Mensuration

TS State Syllabus SSC 10th Class Maths Solutions 10th Lesson Mensuration Exercise 10.1

10th Class Maths 10th Lesson Mensuration Ex 10.1 Textbook Questions and Answers

Question 1.
A joker’s cap is in the form of right circular cone whose base radius is 7 cm and height is 24 cm. Find the area of the sheet required to make 10 such caps.

Answer:

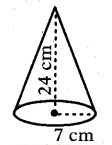
Radius of the cap (r) = 7 cm
Height of the cap (h) = 24 cm
Slant height of the cap (l) = √r²+h²
=√49+576
=√625
=25
∴ I = 25 cm.
Lateral surface area of the cap = Cone = πrl
L.S.A. = 22/7 × 7 × 25 = 550 cm².
∴ Area of the sheet required for 10 caps = 10 x 550 = 5500 cm².

Question 2.
A sports company was ordered to prepare 100 paper cylinders without caps for shuttle cocks. The required dimensions of the cylinder are 35 cm length / height and its radius is 7 cm. Find the required area of thin paper sheet needed to make 100 cylinders.

Answer:

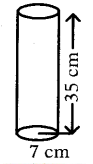
Radius of the cylinder, r = 7 cm
Height of the cylinder, h = 35 cm
T.S.A. of the cylinder with lids at both ends = 2πr(r+h)
= 2 × 22/7 × 7 × (7 + 35)
= 2 × 22/7 × 7 × 42 = 1848 cm².
Area of thin paper required for 100 cylinders = 100 × 1848
= 184800 cm²
= 184800/100×100 m²
= 18.48 m².

Question 3.
Find the volume of right circular cone with radius 6 cm. and height 7 cm.

Answer:

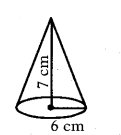
Base radius of the cone (r) = 6 cm.
Height of the cone (h) = 7 cm
Volume of the cone = 1/3 πr²h
= 1/3 × 22/7 × 6 × 6 × 7
= 264 c.c. (Cubic centimeters)
∴Volume of the right circular cone = 264 c.c.

Question 4.
The lateral surface area of a cylinder is equal to the curved surface area of a cone. If their base be the same, find the ratio of the height of the cylinder to slant height of the cone.

Answer:
Base of cylinder and cone be the same.

CSA / LSA of cylinder = 2πrh
CSA of cone = ?rl
The lateral surface area of a cylinder is equal to the curved surface area of cone.
∴ 2πrh = πrl
⇒ h/l=πr/2πr
⇒ h/l = 1/2
∴ h : l = 1 : 2

Question 5.
A self help group wants to manufacture joker’s caps (conical caps) of 3 cm radius and 4 cm height. If the available colour paper sheet is 1000 cm², then how many caps can be manufactured from that paper sheet?

Answer:

Radius of the cap (conical cap) (r) = 3 cm
Height of the cap (h) = 4 cm
Slant height l = √r²+h²
(by Pythagoras theorem)
= √3²+4²
= √9+16
= √25
= 5 cm
C.S.A. of the cap = πrl
= 22/7 × 3 × 5
≃ 47.14 cm²
Number of caps that can be made out of 1000 cm²= 1000/47.14 ≃ 21.27
∴ Number of caps = 21.

Question 6.
A cylinder and cone have bases of equal radii and are of equal heights. Show that their volumes are in the ratio of 3 : 1.

Answer:
Given dimensions are:
Cone:
Radius = r
Height = h
Volume (V) = 13πr²h
Cylinder:
Radius = r
Height = h
Volume (V) = πr²h
Ratio of volumes of cylinder and cone = πr²h : 13πr²h
= 1 : 1/3
= 3 : 1
Hence, their volumes are in the ratio = 3 : 1.

Question 7.
A solid iron rod has cylindrical shape. Its height is 11 cm. and base diameter is 7 cm. Then find the total volume of 50 rods?

Answer:

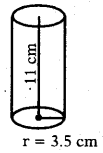
Diameter of the cylinder (d) = 7 cm
Radius of the base (r) = 7/2 = 3.5 cm
Height of the cylinder (h) = 11 cm
Volume of the cylinder V = πr²h
= 22/7 × 3.5 × 3.5 × 11 = 423.5 cm³
∴ Total volume of 50 rods = 50 × 423.5 cm³ = 21175 cm³.

Question 8.
A heap of rice is in the form of a cone of diameter 12 m. and height 8 m. Find its volume? How much canvas cloth is required to cover the heap? (Use π = 3.14)

Answer:

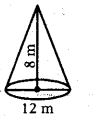
Diameter of the heap (conical) (d) = 12 cm
∴Radius = d/2 = 12/2 = 6 cm
Height of the cone (h) = 8 m
Volume of the cone, V = 1/3πr²h
= 1/3 × 22/7 × 6 × 6 × 8
= 301.71 m³.

Question 9.
The curved surface area of a cone is 4070 cm² and its diameter is 70 cm. What is its slant height?

Answer:
C.S.A. of a cone = πrl = 4070 cm²
Diameter of the cone (d) = 70 cm
Radius of the cone = r = d/2 = 702 = 35 cm
Let its slant height be ‘l’.
By problem,
πrl = 4070 cm²
22/7 × 35 × l = 4070
110 l = 4070
l = 4070/110 = 37 cm
∴ Its slant height = 37 cm.

10th Class Maths 10th Lesson Mensuration Ex 10.2 Textbook Questions and Answers

Question 1.
A toy is in the form of a cone mounted on a hemisphere. The diameter of the base and the height of the cone are 6 cm and 4 cm respectively. Determine the surface area of the toy. (Use π = 3.14)

Answer:

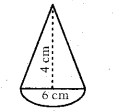
Diameter of the base of the cone d = 6 cm.
∴ Radius of the base of the cone
r = d/2 = 6/2 = 3 cm
Height of the cone = h = 4 cm
Slant height of the cone l = √r²+h²
= √3²+4²
= √9+16
= √25
= 5 cm
∴ C.S.A of the cone = πrl
= 227 × 3 × 5
= 3307 cm²
Radius of the hemisphere = d/2 = 6/2 = 3 cm
C.S.A. of the hemisphere = 2πr²
= 2 × 22/7 × 3 × 3
= 396/7
Hence the surface area of the toy = C.S.A. of cone + C.S.A. of hemisphere
= 330/7 + 396/7
= 726/7 ≃ 103.71 cm².

Question 2.
A solid is in the form of a right circular cylinder with a hemisphere at one end and a cone at the other end. The radius of the common base is 8 cm and the heights of the cylindrical and conical portions are 10 cm and 6 cm respectively. Find the total surface area of the solid. [Use π = 3.14]

Answer:
Total surface area = C.S.A. of the cone + C.S.A. of cylinder + C.S.A of the hemisphere.

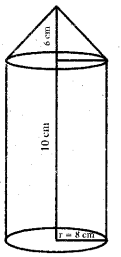
Cone:
Radius (r) = 8 cm
Height (h) = 6 cm
Slant height l =√r²+h²
= √8²+6²
= √64+36
= √100
= 10 cm
C.S.A. = πrl
= 22/7 × 8 × 10
= 1760/7 cm²
Cylinder:
Radius (r) = 8 cm;
Height (h) = 10 cm
C.S.A. = 2πrh
= 2 × 22/7 × 8 × 10
= 3520/7 cm²
Hemisphere:
Radius (r) = 8 cm
C.S.A. = 2πr²
= 2 × 22/7 × 8 × 8
= 2816/7 cm²
∴ Total surface area of the given solid
= 1760/7 + 3520/7 + 2816/7
T.S.A. = 8096/7 = 1156.57 cm².

Question 3.
A medicine capsule is ih the shape of a cylinder with two hemispheres stuck to each of its ends. The length of the capsule is 14 mm. and the width is 5 mm. Find its surface area.

Answer:

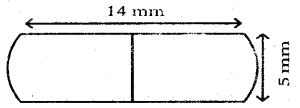
Surface area of the capsule = C.S.A. of 2 hemispheres + C.S.A. of the cylinder
i) Now for Hemisphere:
Radius (r) = d/2 = 5/2 = 2.5 mm
C.S.A of each hemisphere = 2πr²
C.S.A of two hemispheres
= 2 × 2πr² = 4πr²
= 2 × 22/7 × 5/2 × 5/2
= 550/7
= 78.57 mm².

ii) Now for Cylinder:
Length of capsule = AB =14 mm
Then height (length) cylinder part = 14 - 2(2.5)
h = 14 - 5 = 9 mm
Radius of cylinder part (r) = 5/2
Now C.S.A of cylinder part = 2πrh
= 2 × 22/7 × 5/2 × 9
= 900/7
= 141.428 mm²
Now total surface area of capsule
= 78.57 + 141.43 = 220 mm²

Question 4.
Two cubes each of volume 64 cm³ are joined end to end together. Find the surface area of the resulting cuboid.

Answer:
Given, volume of the cube.
V = a³ = 64 cm³
∴ a³ = 4 × 4 × 4 = 4³ , Hence a = 4 cm
When two cubes are added, the length of cuboid = 2a = 2 × 4 = 8 cm,
breadth = a = 4 cm.
height = a = 4 cm is formed.

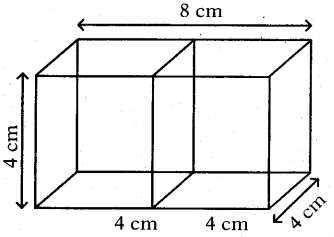
∴ T.S.A. of the cuboid
= 2 (lb + bh + lh)
= 2(8 × 4 + 4 × 4 + 8 × 4)
= 2(32 + 16 + 32)
= 2 × 80
= 160 cm²
∴The surface area of resulting cuboid is 160 cm².

Question 5.
A storage tank consists of a circular cylinder with a hemisphere stuck on either end. If the external diameter of the cylinder be 1.4 m. and its length be 8 m. Find the cost of painting it on the outside at rate of Rs. 20 per m².

Answer:
Total surface area of the tank = 2 × C.S.A. of hemisphere + C.S.A. of cylinder.

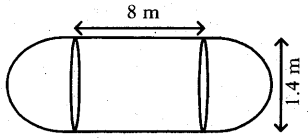
Hemisphere:
Radius (r) = d/2 = 1.4/2 = 0.7 m
C.S.A. of hemisphere = 2πr²
= 2 × 22/7 × 0.7 × 0.7
= 3.08 m².
2 × C.S.A. = 2 × 3.08 m² = 6.16 m²
Cylinder:
Radius (r) = d/2 = 1.4/2 = 0.7 m
Height (h) = 8 m
C.S.A. of the cylinder = 2πrh
= 2 × 22/7 × 0.7 × 8
= 35.2 m²
∴ Total surface area of the storage tank = 35.2 + 6.16 = 41.36 m²
Cost of painting its surface area @ Rs. 20 per sq.m, is
= 41.36 × 20 = Rs. 827.2.

Question 6.
A hemisphere is cut out from one face of a cubical wooden block such that the diameter of the hemisphere is equal to the length of the cube. Determine the surface area of the remaining solid.

Answer:
Let the length of the edge of the cube = a units

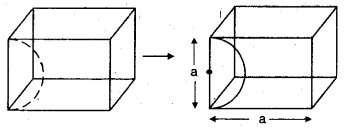
T.S.A. of the given solid = 5 × Area of each surface + Area of hemisphere
Square surface:
Side = a units
Area = a² sq. units
5 × square surface = 5a² sq. units
Hemisphere:
Diameter = a units;
Radius = a/2
C.S.A. = 2πr²
= 2π(a/2)²
= 2πa²/4 = πa²/2 sq. units
Total surface area = 5a² + πa²/2 = a²(5+π/2) sq. units.

Question 7.
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in the figure. If the height of the cylinder is 10 cm and its base radius is of 3.5 cm, find the total surface area of the article.

Answer:
Surface area of the given solid = C.S.A. of the cylinder + 2 × C.S.A. of hemisphere.
If we take base = radius
Cylinder:
Radius (r) = 3.5 cm
Height (h) = 10 cm
C.S.A. = 2πrh
= 2 × 22/7 × 3.5 × 10
= 220 cm²
Hemisphere:
Radius (r) = 3.5 cm
C.S.A. = 2πr²
= 2 × 22/7 × 3.5 × 3.5
= 77 cm²
2 × C.S.A. = 2 × 77 = 154 cm²
∴ T.S.A. = 220 + 154 = 374 cm²

10th Class Maths 11th Lesson Trigonometry Ex 11.3 Textbook Questions and Answers

Question 1.
Evaluate:

i) tan36°/cot54°
Answer:
Given that tan36°/cot54°
= tan36°/cot(90°?36°) [∵ cot (90 - θ) = tan θ]
= tan36°/tan36°
= 1
ii) cos 12° - sin 78°
Answer:
Given that cos 12° - sin 78°
= cos 12° - sin(90° - 12°) [∵ sin (90 - θ) = cos θ]
= cos 12° - cos 12° = 0
iii) cosec 31° - sec 59°
Answer:
Given that cosec 31° - sec 59°
= cosec 31° - sec (90° - 31°) [∵ sec (90 - θ) = cosec θ]
= cosec 31° - cosec 31° = 0
iv) sin 15° sec 75°
Answer:
Given that sin 15° sec 75°
= sin 15° . sec (90° - 15°)
= sin 15° . cosec 15° [∵ sec (90 - θ) = cosec θ]
= sin 15° . 1/sin15° [∵ cosec θ = 1/sinθ]
= 1
v) tan 26° tan 64°
Answer:
Given that tan 26° tan 64°
= tan 26° . tan (90° - 26°)
= tan 26° . cot 26° [∵ tan (90 - θ) = cot θ]
= tan 26° . 1/tan26° [∵ cot θ = 1/tanθ]
= 1

Question 2.
Show that

i) tan 48° tan 16° tan 42° tan 74° = 1
Answer:
L.H.S. = tan 48° tan 16° tan 42° tan 74°
= tan 48°. tan 16° . tan(90° - 48°) . tan(90° - 16°)
= tan 48° . tan 16° . cot 48° . cot 16° [∵ tan (90 - θ) = cot θ]
= tan 48° . tan 16° . 1/tan48° . 1/tan16° [∵ cot θ = 1t/anθ]
= 1 = R.H.S.
∴ L.H.S. = R.H.S.
ii) cos 36° cos 54° - sin 36° sin 54° = 0
Answer:
L.H.S. = cos 36° cos 54° - sin 36° sin54°
= cos (90° - 54°) . cos (90° - 36°) - sin 36° . sin 54° [∵ cos (90 - θ) = sin θ]
= sin 54° . sin 36° - sin 36° . sin 54°
= 0 = R.H.S.
∴ L.H.S. = R.H.S.

Question 3.
If tan 2A = cot (A - 18°), where 2A is an acute angle. Find the value of A.

Answer:
Given that tan 2A = cot (A - 18°)
⇒ cot (90°- 2A) = cot (A - 18°) [∵ tan θ = cot (90 - θ)]
⇒ 90° - 2A = A - 18°
⇒ 108° = 3A
⇒ A = 108°/3 = 36°
Hence the value of A is 36°.

Question 4.
If tan A = cot B where A and B. are acute angles, prove that A + B = 90°.

Answer:
Given that tan A = cot B
⇒ cot (90° - A) = cot B [∵ tan θ = cot (90 - θ)]
⇒ 90° - A = B
⇒ A + B = 90°

Question 5.
If A, B and C are interior angles of a triangle ABC, then show that tan(A+B/2)=cot C/2

Answer:
Given A, B and C are interior angles of right angle triangle ABC then A + B + C = 180°.
On dividing the above equation by 2 on both sides, we get 180°

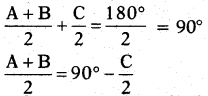
On taking tan ratio on both sides

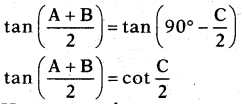
Hence proved.

Question 6.
Express sin 75° + cos 65° in terms of trigonometric ratios of angles between 0° and 45°.

Answer:
We have sin 75° + cos 65°
= sin (90° - 15°) + cos (90° - 25°)
= cos 15° + sin 25° [∵ sin (90 - θ) = cos θ and cos (90 - θ) = sin θ]

10th Class Maths 11th Lesson Trigonometry Ex 11.4 Textbook Questions and Answers

Question 1.
Evaluate the following:

i) (1 + tan θ + sec θ) (1 + cot θ - cosec θ)
Answer:
Given (1 + tan θ + sec θ) (1 + cot θ - cosec θ)

ii) (sin θ + cos θ)² + (sin θ - cos θ)²
Answer:
Given (sin θ + cos θ)2 + (sin θ - cos θ)²
= (sin² θ + cos² θ + 2 sin θ cos θ) + (sin² θ + cos² θ - 2 sin θ cos θ) [∵ (a + b)² = a² + b² + 2ab
(a - b)² = a² + b² - 2ab]
= 1 + 2 sin θ cos θ + 1 - 2 sin θ cos θ [∵ sin² θ + cos² θ = 1]
= 1 + 1
= 2
iii) (sec² θ - 1) (cosec² θ - 1)
Answer:
Given (sec² θ - 1) (cosec² θ - 1)
= tan² θ × cot2 θ [∵ sec² θ - tan² θ = 1; cosec² θ - cot² θ = 1]
= tan² θ × 1/tan²θ = 1

Question 2.
Show that (cosec θ - cot θ)² = 1?cosθ/1+cosθ

Answer:

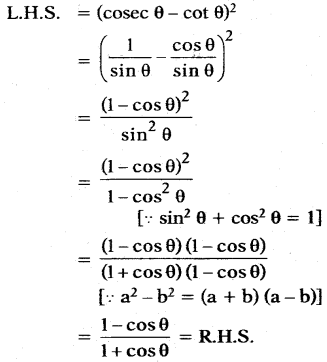
Question 3.
Show that √1+sinA/1?sinA = sec A + tan A

Answer:
Given that L.H.S. = √1+sinA/1?sinA
Rationalise the denominator, rational factor of 1 - sin A is 1 + sin A.

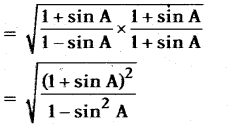
[∵ (a + b)(a + b) = (a + b)²]; (a - b)(a + b) = a² - b²]
= √(1+sinA)²/cos²A
= 1+sinA/cosA
= 1/cosA + sinA/cosA
= sec A + tan A = R.H.S.

Question 4.
Show that 1?tan²A/cot²A?1 = tan² A

Answer:

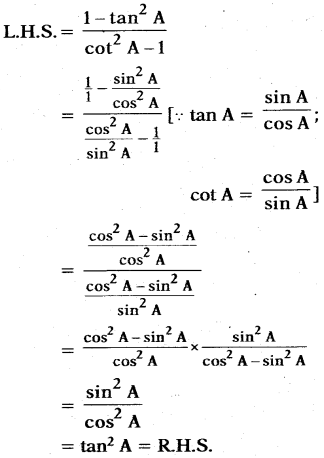
Question 5.
Show that 1/cosθ - cos θ = tan θ - sin θ.

Answer:

Question 6.
Simplify sec A (1 - sin A) (sec A + tan A)

Answer:
L.H.S. = sec A (1 - sin A) (sec A + tan A)
= (sec A - sec A . sin A) (sec A + tan A)
= (sec A - 1/cosA . sin A) (sec A + tan A)
= (sec A - tan A) (sec A + tan A)
= sec² A - tan² A [∵ sec² A - tan² A = 1]
= 1

Question 7.
Prove that (sin A + cosec A)² + (cos A + sec A)² = 7 + tan² A + cot² A

Answer:
L.H.S. = (sin A + cosec A)² + (cos A + sec A)²
= (sin² A + cosec² A + 2 sin A . cosec A) + (cos² A - sec² A + 2 cos A . sec A) [∵ (a + b)² = a² + b² + ²ab]
= (sin² A + cos² A) + cosec² A + ² sin A . 1/sinA + sec² A + 2 cos A . 1/cosA
[∵ 1/sinA = cosec A; 1/cosA = sec A]
= 1 +(1 + cot² A) + 2 + (1 + tan² A) + 2
[∵ sin² A + cos² A = 1; cosec² A = 1 + cot² A; sec² A = 1 + tan² A]
= 7 + tan² A + cot² A
= R.H.S.

Question 8.
Simplify (1 - cos θ) (1 + cos θ) (1 + cot² θ)

Answer:
Given that
(1 - cos θ) (1 + cos θ) (1 + cot² θ)
= (1 - cos² θ) (1 + cot² θ)
[∵ (a - b) (a + b) = a² - b²]
= sin² θ. cosec² θ [∵ 1 - cos² θ = sin² θ; 1 + cot² θ = cosec² θ]
= sin² θ . 1/sin²θ [∵ cosec θ = 1/sinθ]
= 1

Question 9.
If sec θ + tan θ = p, then what is the value of sec θ - tan θ?

Answer:
Given that sec θ + tan θ = p ,
We know that sec² θ - tan² θ = 1
sec² θ - tan² θ = (sec θ + tan θ) (sec θ - tan θ)
= p (sec θ - tan θ)
= 1 (from given)
⇒ sec θ - tan θ = 1/p

Question 10.
If cosec θ + cot θ = k, then prove that cos θ = k²-1/k²+1

Answer:
Method-I:
Given that cosec θ + cot θ = k
R.H.S. = k²-1/k²+1

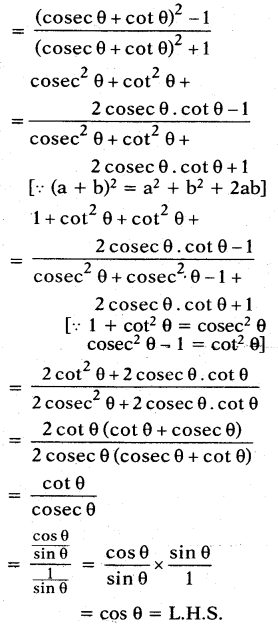
Method - II:
Given that cosec θ + cot θ = k ....(1)
We know that cosec² θ - cot² θ = 1
⇒ (cosec θ + cot θ) (cosec θ - cot θ) = 1 [∵ a² - b² = (a -b)(a + b)]
⇒ k (cosec θ - cot θ) = 1
⇒ (cosec θ - cot θ) = 1/k
By solving (1) and (2)

According to identity cos² θ + sin² θ = 1

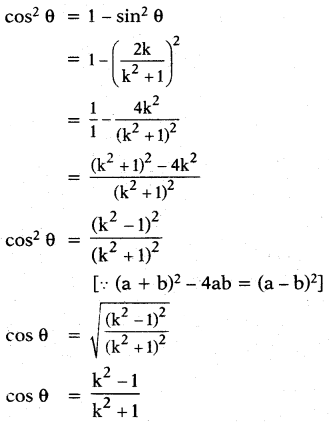
Hence proved.