Maths Class 10th Surface Areas and Volumes

NCERT CBSE for Class 10 Maths Chapter 13 Surface Areas & Volumes

Surface Areas & Volumes Class 10 Important Questions Very Short Answer (1 Mark)

Question 1.
A sphere of diameter 18 cm is dropped into a cylindrical vessel of diameter 36 cm, partly filled with water. If the sphere is completely submerged, then calculate the rise of water level (in cm).
Year of Question: (2011D)

Solution:
Volume of Cylinder = Volume of Sphere

Question 2.
Find the number of solid spheres, each of diameter 6 cm that can be made by melting a solid metal cylinder of height 45 cm and diameter 4 cm.
Year of Question: (2014D)

Solution:
Number of solid spheres

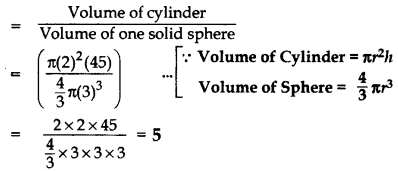
Question 3.
A solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. Find the ratio of the volume of the smaller cone to the whole cone.
Year of Question: (2012OD)

Solution:
Since the cone is cut from the middle,

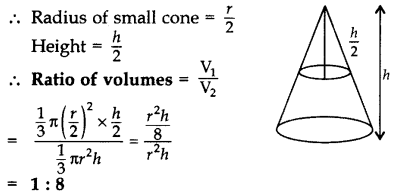
Question 4.
Volume and surface area of a solid hemisphere are numerically equal. What is the diameter of hemisphere?
Year of Question: (2017D)

Solution:
Volume of hemisphere = Surface area of hemisphere .[Given
2/3πr³ = 2πr² ⇒ 1/3r = 1
r = 3
∴ Diameter of hemisphere = 27 = 2(3) = 6 cm

Surface Areas and Volumes Class 10 Important Questions Short Answer-I (2 Marks)

Question 5.
If the total surface area of a solid hemisphere is 462 cm², find its volume. [Take π = 22/7]
Year of Question: (2014OD)

Solution:
Total surface area of hemisphere = 462 cm²

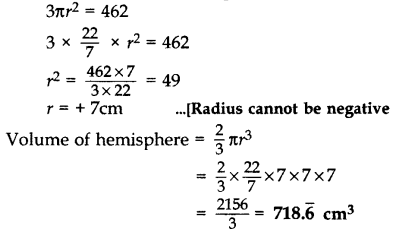
Surface Areas and Volumes Class 10 Important Questions Short Answer-II (3 Marks)

Question 6.
Two cubes, each of side 4 cm are joined end to end. Find the surface area of the resulting cuboid.
Year of Question: (2011D)

Solution:
Length of resulting cuboid, I = 2(4) = 8 cm
Breadth of resulting cuboid, b = 4 cm
Height of resulting cuboid, h = 4 cm
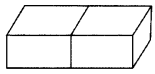Surface area of resulting cuboid
= 2(lb + bh +hl) = 2 [8(4) + 4(4) + 4(8)]
= 2 (32 + 16 + 32) = 2 (80) = 160 cm²

Question 7.
The radii of the circular ends of a bucket of height 15 cm are 14 cm and r ?m (r < 14 cm). If the volume of bucket is 5390 cm³, then find the value of r. [Use π = 22/7]
Year of Question: (2011D)

Solution:
Here h = 15 cm, R = 14 cm,‘r’ = r cm
Volume of bucket = 5390 cm³

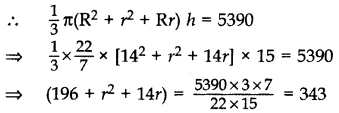
⇒ r² + 14r + 196 - 343 = 0
⇒ r² + 14r - 147 = 0 = r² + 21r - 77 - 147 = 0
⇒ r(r + 21) - 7 (r + 21) = 0
⇒ (r + 21) (r - 7) = 0
⇒ r + 21 = 0 or r - 7 = 0 =
⇒ r = -21 (rejected) or r = 7
..[∵ Radius cannot be negative
∴ Radius, r = 7 cm

Question 8.
Two cubes each of volume 27 cm³ are joined end to end to form a solid. Find the surface area of the resulting cuboid.
Year of Question: (2011OD)

Solution:
Volume of a cube = 27 cm³
⇒ (Side)³ = (3)³ ∴ Side = 3 cm
Length of resulting cuboid, l = 2 × 3 = 6 cm
Breadth of resulting cuboid, b = 3 cm
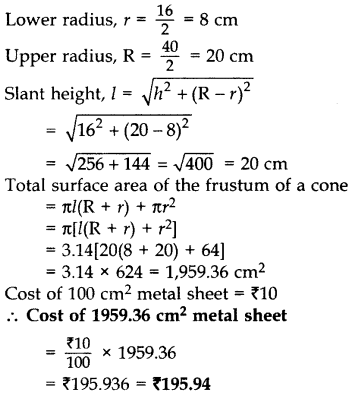
Height of resulting cuboid, h = 3 cm
Surface area of resulting cuboid = 2(lb + bh + hl)
= 2(6 × 3 + 3 × 3 + 3 × 6)
= 2(18 + 9 + 18) = 2(45) = 90 cm²

Question 9.
The sum of the radius of base and height of a solid right circular cylinder is 37 cm. If the total surface area of the solid cylinder is 1628 sq. cm, find the volume of the cylinder. (Use π = 22/7)
Year of Question: (2016D)

Solution:
Let the radius and height of cylinder be r and h respectively
r + h = 37 cm .(i) [Given
Total surface area of cylinder = 1,628 cm²
2πr(r + h) = 1,628
⇒ 2πr(37) = 1,628

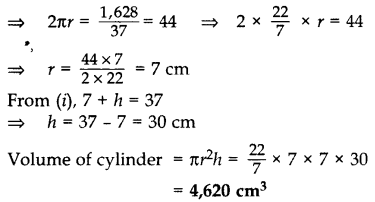
Question 10.
A right circular cone of radius 3 cm, has a curved surface area of 47.1 cm². Find the volume of the cone. (Use π = 3.14)
Year of Question: (2016D)

Solution:
C.S. Area of cone = 47.1 cm² .[Given
.[Here, r = 3 cm
πrl = 47.1 = (3.14)(3)l = 47.1
Slant height, l = 47.1/3.14(3) = 5 cm
⇒ r² + h² = l² .[Pythagoras’ theorem
⇒ (3)² + h² = (5)² ⇒ h² = 25 - 9 = 16
⇒ h = +4 cm
Volume of the cone = 1/3 πr²h = 13 (3.14)(3)²(4)
= 3.14(12) = 37.68 cm3

Question 11.
An icecream seller sells his icecreams in two ways:
Year of Question: (2012OD)

(A) In a cone of r = 5 cm, h = 8 cm
(B) In a cup in shape of cylinder with r = 5 cm, h = 8 cm

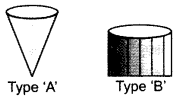
He charges the same price for both but prefers to sell his icecream in a cone.
(a) Find the volume of the cone and the cup.
(b) Which out of the two has more capacity?
Solution:
Volume of type ‘A’
Volume of cone + Volume of hemisphere

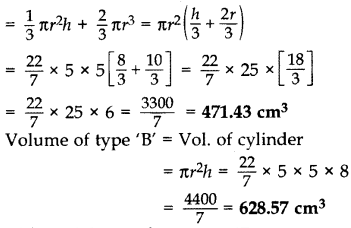
(a) ∴ Volume of a cone = 471.43 cm³
Volume of a cup = 628.57 cm³
(b) Cup has more capacity.

Question 12.
A vessel is in the form of a hemispherical bowl surmounted by a hollow cylinder of same diameter. The diameter of the hemispherical bowl is 14 cm and the total height of the vessel is 13 cm. Find the total (inner) suface area of the vessel. (Use π = 22/7)
Year of Question: (2013D)

Solution:
r = 14/2 = 7 cm
Inner surface area of the vessel = C.S. area of Hemi-sphere + C.S. area of Cylinder
= 2πr² + 2πrh = 2πr(r + h) .C.S. area = curved surface area
= 2 × 22/7 × 77 + 6) = 44 × 13 = 572 cm²

Question 13.
A solid wooden toy is in the form of a hemisphere surmounted by a cone of same radius. The radius of hemisphere is 3.5 cm and the total wood used in the making of toy is 166 5/6 cm³. Find the height of the toy. Also, find the cost of painting the hemispherical part of the toy at the rate of ₹10 per cm². [Use π = 22/7]
Year of Question: (2015D)

Solution:
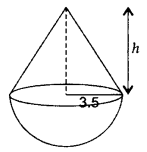Let the height of cone = h
Radius of cone = Radius of hemisphere = r = 3.5 cm
Volume of solid wooden toy = Volume of hemisphere + Volume of cone

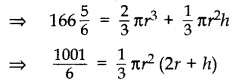
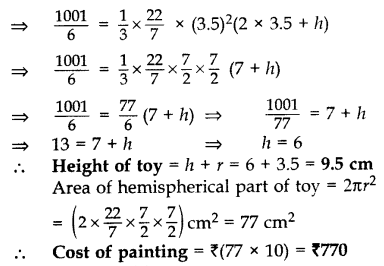
Question 14.
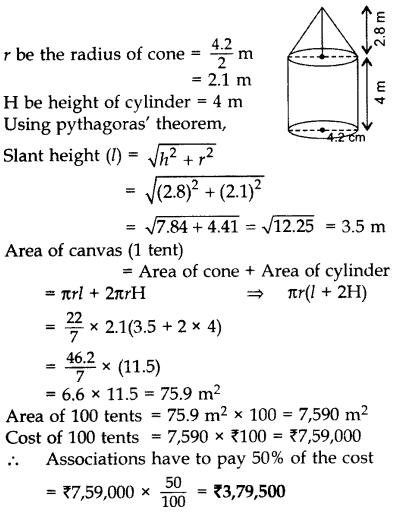
Due to sudden floods, some welfare associations jointly requested the government to get 100 tents fixed immediately and offered to contribute 50% of the cost. If the lower part of each tent is of the form of a cylinder of diameter 4.2 m and height 4 m with the conical upper part of same diameter but of height 2.8 m, and the canvas to be used costs 100 per sq. m, find the amount, the associations will have to pay. (Use π = 22/7)
Year of Question: (2015OD)

Solution:
Let l be the slant height of cone
h be the height of cone = 2.8 m

Question 15.
A cubical block of side 10 cm is surmounted by a hemisphere. What is the largest diameter that the hemisphere can have? Find the cost of painting the total surface area of the solid so formed, at the rate of ₹5 per 100 sq. cm. (Use π = 3.14)
Year of Question: (2015OD)

Solution:
Let the side of cuboidal block (a) = 10 cm
Let the radius of hemisphere be r.
Side of cube = Diameter of hemisphere Largest possible diameter of hemisphere = 10 cm
∴ Radius, r = 102 = 5 cm
Total surface area = Total surface area of cube + Curved surface area of hemisphere- Area of base

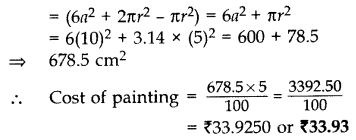
Question 16.
In Figure, is a decorative block, made up of two solids-a cube and a hemisphere. The base of the block is a cube of side 6 cm and the hemisphere fixed on the top has a diameter of 3.5 cm. Find the total surface area of the block. (Use π= 22/7)
Year of Question: (2016D)

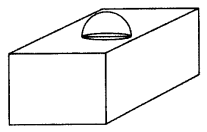
Solution:
Total surface area of the block
= Total surface area of cube + C.S. Area of hemisphere - Area of circle
= 6(side)² + 2πr² - πr²
= 6(side)² + πr²

Question 17.
A conical vessel, with base radius 5 cm and height 24 cm, is full of water. This water is emptied into a cylindrical vessel of base radius 10 cm. Find the height to which the water will rise in the cylindrical vessel. (Use π= 22/7)
Year of Question: (2016D)

Solution:
Let r and I be the radius and height of conical vessel i.e., 5 cm and 24 cm respectively.
Let H be the height of rise in water in cylindrical vessel and R be the radius, i.e., 10 cm.
Volume of water in cylindrical vessel = Volume of water in conical vessel

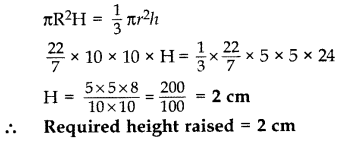
Question 18.
A milkman was serving his customers using two types of mugs A and B of inner diameter 5 cm to Mug‘A Mug‘B’ serve the customers. The height of the mugs is 10 cm.
Year of Question: (2012D)

He decided to serve the customers in ‘B’ type of mug.
(a) Find the volume of the mugs of both types.
(b) Which mathematical concept is used in the above problem?

Solution:
(a) Let the radius of cylinder, hemi-sphere and cone be r ?m
Let the height of cylinder and cone h₁ and h₂ respectively.

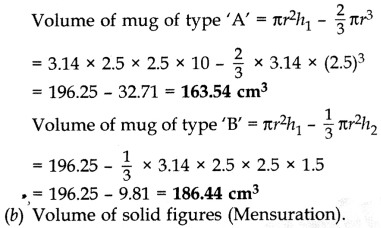
Question 19.
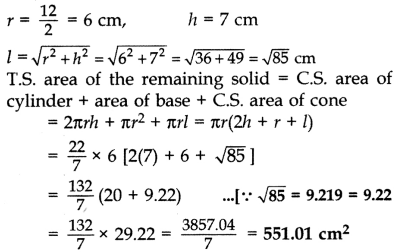
From a solid cylinder of height 7 cm and base diameter 12 cm, a conical cavity of same height and same base diameter is hollowed out. Find the total surface area of the remaining solid. [Use π = 22/7]
Year of Question: (2012D)

Solution:

Question 20.
A wooden toy was made by scooping out a hemisphere of same radius from each end of a solid cylinder. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the volume of wood in the toy. [Use π = 22/7]
Year of Question: (2013D)

Solution:
Here r = 3.5 cm = 72 cm, h = 10 cm
Volume of wood in the toy = Volume of cylinder - 2(Vol. of hemisphere)

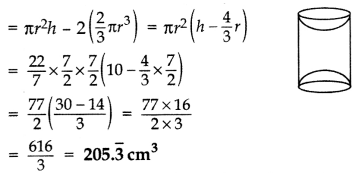
Question 21.
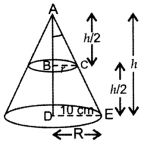
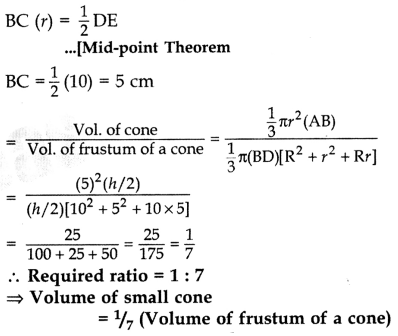
A solid cone of base radius 10 cm is cut into two parts through the mid-point of its height, by a plane parallel to its base. Find the ratio in the volumes of the two parts of the cone.
Year of Question: (2013OD)

Solution:
Let BC = r ?m and
DE = R = 10 cm
B and C are the mid-points of AD and AE respectively. .[Given

Question 22.
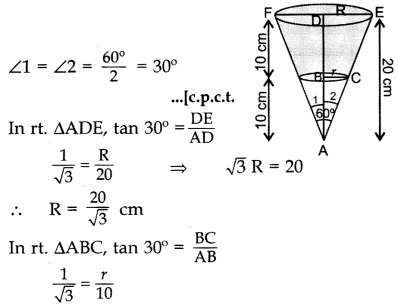
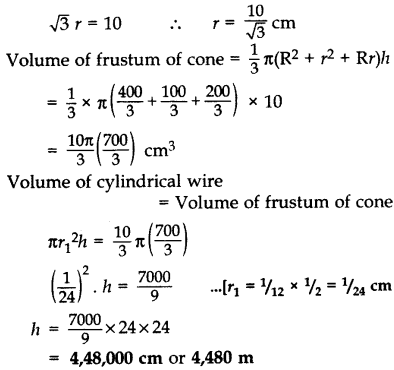
A solid metallic right circular cone 20 cm high and whose vertical angle is 60°, is cut into two parts at the middle of its height by a plane parallel to its base. If the frustum so obtained be drawn into a wire of diameter 1/12 cm, find the length of the wire.
Year of Question: (2014D)

Solution:
A solid cone AFE has been cut by BC ‖| FE and
AD ⊥ FE.
∆ADF = ∆ADE (SSS)

Question 23.
The largest possible sphere is carved out of a wooden solid cube of side 7 cm. Find the volume of the wood left. (Use π = 22/7]
Year of Question: (2014OD)

Solution:
Volume of the wood left = Volume of cube - Volume of sphere

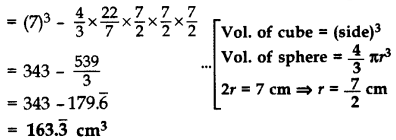
Question 24.
In Figure, from the top of a solid cone of height 12 cm and base radius 6 cm, a cone of height 4 cm is removed by a plane parallel to the base. Find the total surface area of the remaining solid. (Use π = 22/7 and √3 = 2.236)
Year of Question: (2015D)

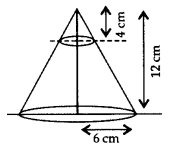
Solution:

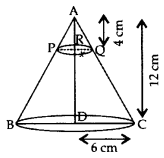
Let AR = 4 cm,
RD = 12 - 4 = 8 cm
Height of frustum (RD),
h = 8 cm Lower radius of frustum (DC),
r₁ = 6 cm
We know, ∆ARQ ≅ ∆ADC

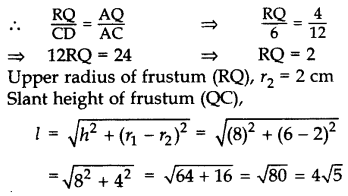
Question 25.
In Figure, from a cuboidal solid metallic block, of dimensions 15 cm × 10 cm × 5 cm, a cylindrical hole of diameter 7 cm is drilled out. Find the surface area of the remaining block. (Use π = 22/7)
Year of Question: (2015D)

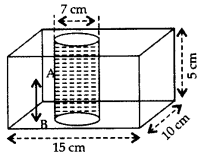
Solution:
Let the length, breadth, height of cuboidal block be 15 cm, 10 cm and 5 cm respectively.
Total surface area of solid cuboidal block
= 2(lb + bh + lh)
= 2(15 × 10 + 10 × 5 + 15 × 5) cm²
= 2(150 + 50 + 75) = 2(275) = 550 cm²
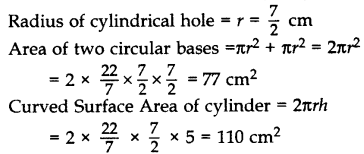Required area = (Area of cuboidal block - Area of two circular bases + Area of cylinder)
= (550 + 110 - 77) cm² = 583 cm²

Question 26.
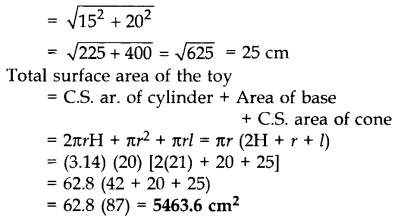
A toy is in the shape of a solid cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 21 cm and 40 cm respectively, and the height of cone is 15 cm, then find the total surface area of the toy. [π = 3.14, be taken]
Year of Question: (2011D)

Solution:
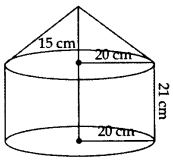Height of cylinder, H = 21 cm
Height of cone, h = 15 cm
Common radius,

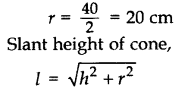
Question 27.
A cone of height 20 cm and radius of base 5 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the diameter of the sphere.
Year of Question: (2011OD)

Solution:
Volume of the Sphere = Volume of Cone
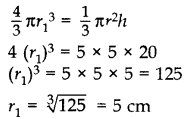∴ Diameter of the sphere = 2r₁ = 2(5) = 10 cm

Question 28.
A cylindrical bucket, 32 cm high and with radius of base 18 cm, is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 24 cm, then find the radius and slant height of the heap.
Year of Question: (2012D)

Solution:
Let x be radius of the conical heap.
Volume of conical heap = Volume of cyl. bucket

Question 29.
A solid metallic sphere of diameter 8 cm is melted and drawn into a cylindrical wire of uniform width. If the length of the wire is 12 m, find its width.
Year of Question: (2013OD)

Solution:
Vol. of cylindrical wire = Volume of solid sphere

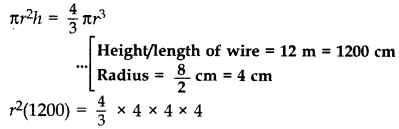
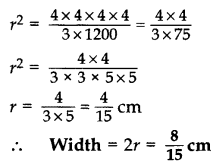
Question 30.
A well of diameter 4 m is dug 21 m deep. The earth taken out of it has been spread evenly all around it in the shape of a circular ring of width 3 m to form an embankment. Find the height of the embankment.
Year of Question: (2016D)

Solution:
Radius of well, r = 4/2 = 2 m
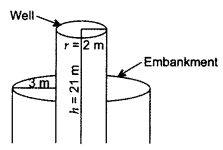Radius of embankment, R = 2 + 3 = 5 m
Height of the well, h = 21 m
Required height raised

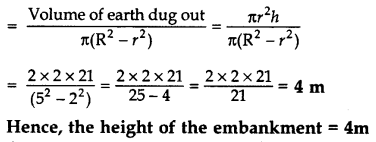
Question 31.
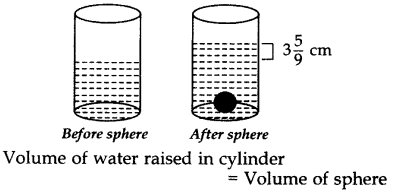
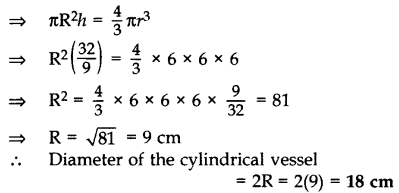
A sphere of diameter 12 cm, is dropped in a right circular cylindrical vessel, partly filled with water. If the sphere is completely submerged in water, the water level in the cylindrical vessel rises by 3 5/9 cm. Find the diameter of the cylindrical vessel.
Year of Question: (2016D)

Solution:
Radius of sphere, r = 12/2 = 6 cm
Let h be the height of risen water level i.e., 3 5/9 cm or 32/9 cm and R be the radius of cylindrical vessel.

Question 32.
A solid sphere of radius 10.5 cm is melted and recast into smaller solid cones, each of radius 3.5 cm and height 3 cm. Find the number of cones so formed. (Use π= 22/7)
Year of Question: (2012OD)

Solution:

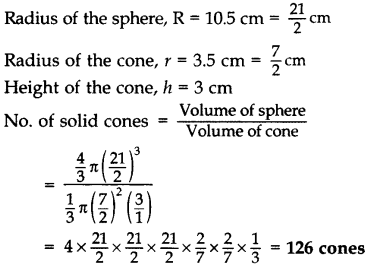
Question 33.
The 3/4 th part of a conical vessel of internal radius 5 cm and height 24 cm is full of water. The water is emptied into a cylindrical vessel with internal radius 10 cm. Find the height of water in cylindrical vessel.
Year of Question: (2017D)

Solution:

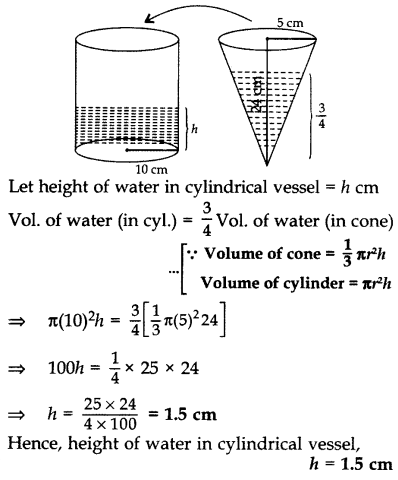
Question 34.
504 cones, each of diameter 3.5 cm and height 3cm, are melted and recast into a metallic sphere. Find the diameter of the sphere and hence find its surface area. (Use π = 22/7)
Year of Question: (2015OD)

Solution:
Let the radius of sphere (R) = ?
Let the radius of cone (r) = 3.5/2 = 35/20
Let the height of cone (h) = 3 cm
Volume of metal in 1 cone = 1/3 πr²h
Volume of metal in 504 cones

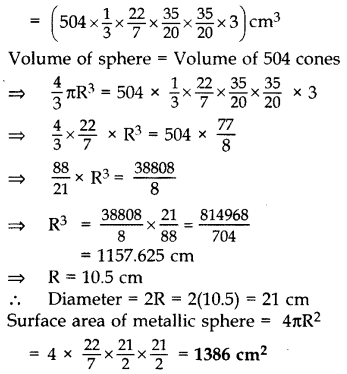
Question 35.
A farmer connects a pipe of internal diameter 20 cm from a canal into a cylindrical tank which is 10 m in diameter and 2 m deep. If the water flows through the pipe at the rate of 4 km per hour, in how much time will the tank be filled completely?
Year of Question: (2014D)

Solution:

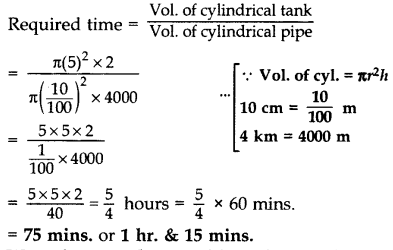
Question 36.
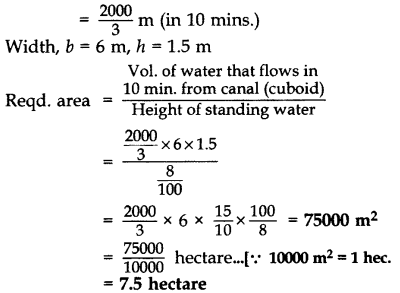
Water in a canal, 6 m wide and 1.5 m deep, is flowing at a speed of 4 km/h. How much area will it irrigate in 10 minutes, if 8 cm of standing water is needed for irrigation?
Year of Question: (2014OD)

Solution:
Speed of water = 4 km/hr
Canal, l = 4 × 10/60 = 2/3 km

Question 37.
A hemispherical bowl of internal diameter 36 cm contains liquid. This liquid is filled into 72 cylindrical bottles of diameter 6 cm. Find the height of the each bottle, if 10% liquid is wasted in this transfer.
Year of Question: (2015OD)

Solution:
Let the radius of hemispherical bowl,
(R) = 36/2 = 18 cm
Let the radius of cylindrical bottle (r) = 62 = 3 cm
Let the height of cylindrical bottle be h = ?
Vol. of liquid in the hemispherical bowl = 23πR³

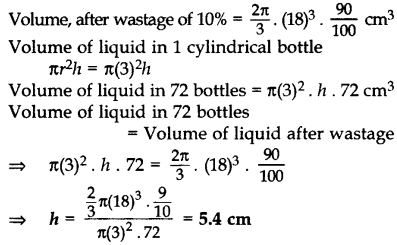
Question 38.
An open metal bucket is in the shape of a frustum of a cone of height 21 cm with radii of its lower and upper ends as 10 cm and 20 cm respectively. Find the cost of milk which can completely fill the bucket at ₹30 per litre. [Use π = 22/7]
Year of Question: (2011OD)

Solution:
Here h = 21 cm, r = 10 cm, R = 20 cm

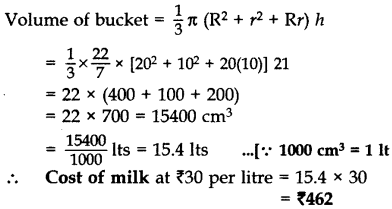
Question 39.
A toy is in the form of a cone mounted on a hemisphere of same radius 7 cm. If the total height of the toy is 31 cm, find its total surface area. (Useπ = 22/7)
Year of Question: (2013OD)

Solution:

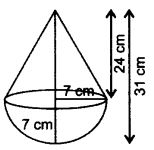
Radius = 7 cm
Vertical height of cone,
h = 31 - 7 = 24 cm
Slant height,

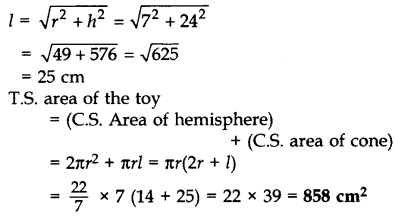
Question 40.
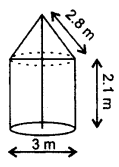
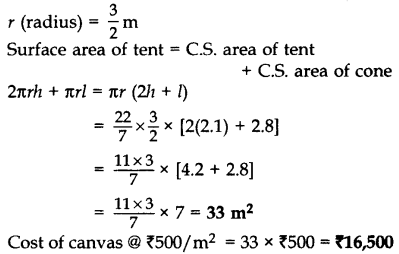
In Figure, a tent is in the shape of a cylinder surmounted by a conical top of same diameter. If the height and diameter of cylindrical part are 2.1 m and 3 m respectively and the slant height of conical part is 2.8 m, find the cost of canvas needed to make the tent if the canvas is available at the rate of ₹500/sq. metre. (Use π = 22/7]
Year of Question: (2016OD)

Solution:

Surface Areas and Volumes Class 10 Important Questions Long Answer (4 Marks)

Question 41.
A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 7 cm and the height of the cone is equal to its diameter. Find the volume of the solid. [Use π = 22/7]
Year of Question: (2012D)

Solution:
Radius, r = 7 cm
Height of cone, h = 2(7) = 14 cm
Volume of solid = Vol. of hemisphere + Volume of cone

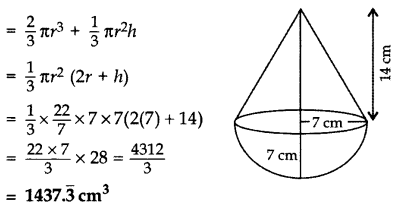
Question 42.
A hemispherical tank, full of water, is emptied by a pipe at the rate of 25/7 litres per sec. How much time will it take to empty half the tank if the diameter of the base of the tank is 3 m?
Year of Question: (2012OD)

Solution:

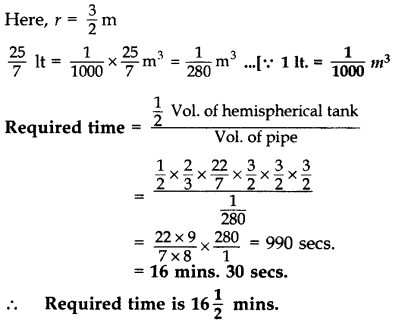
Question 43.
Water is flowing through a cylindrical pipe, of internal diameter 2 cm, into a cylindrical tank of base radius 40 cm, at the rate of 0.4 m/s. Determine the rise in level of water in the tank in half an hour.
Year of Question: (2013D)

Solution:
Radius of tank, r₁ = 40 cm
Internal radius of cylindrical pipe, r₂ = 2/2 = 1 cm
Let the height of rises water, h₁ = ?
Length of water flow in 1 second = 0.4 m
= 4/10 × 100 = 40 cm
∴ Length of water flow in 30 minutes, h₂
= 40 × 60 × 30 = 72,000 cm
Volume of water in cylinder tank
= Volume of water flow from cylindrical pipe in half an hour
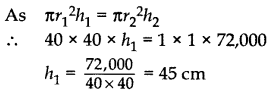∴ Level of water in cylinder tank rises in half an hour, h₁ = 45 cm

Question 44.
150 spherical marbles, each of diameter 1.4 cm, are dropped in a cylindrical vessel of diameter 7 cm containing some water, which are completely immersed in water. Find the rise in the level of water in the vessel.
Year of Question: (2014OD)

Solution:

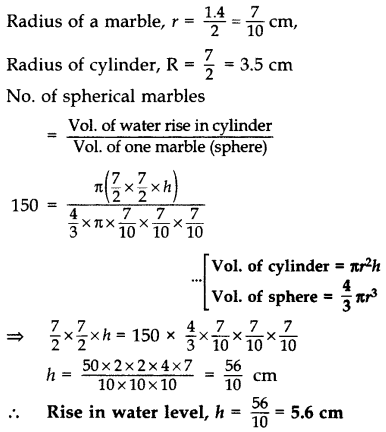
Question 45.
From a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Take π= 22/7)
Year of Question: (2014D)

Solution:

Question 46.
Water is flowing at the rate of 2.52 km/hr. through a cylindrical pipe into a cylindrical tank, the radius of whose base is 40 cm. If the increase in the level of water in the tank, in half an hour is 3.15 m, find the internal diameter of the pipe.
Year of Question: (2015D)

Solution:
Let r be the internal radius of the pipe.
Radius of base of tank, R = 40 cm
= 40/100=2/5 m
Level of water raised in the tank (H) = 3.15 m
If the flow rate in an hour = 2.52 km = 2520 m
Then, the flow rate in half an hour
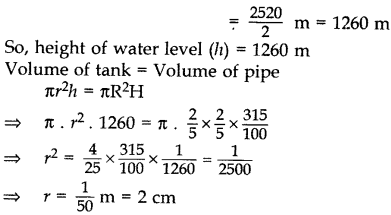∴ Internal diameter of pipe = 2r = 2 × 2 = 4 cm
∴Internal diameter of pipe = 4 cm

Question 47.
From each end of a solid metal cylinder, metal was scooped out in hemispherical form of same diameter. The height of the cylinder is 10 cm and its base is of radius 4.2 cm. The rest of the cylinder is melted and converted into a cylindrical wire of 1.4 cm thickness. Find the length of the wire. [Use π = 22/7]
Year of Question: (2015OD)

Solution:
Let h be the height of cylinder = 10 cm
Let r be the radius of cylinder = 4.2 cm
Radius of cylinder
= Radius of hemispherical metal = r = 4.2 cm ..[Given
Total Volume of cylinder = πr²h

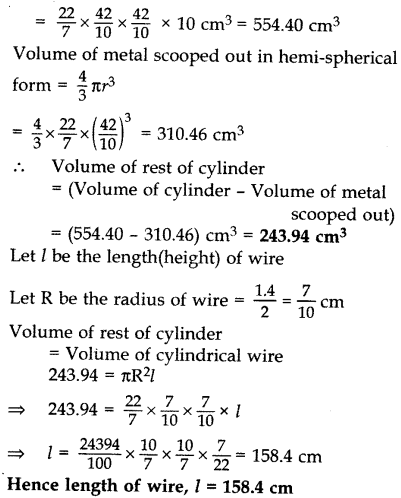
Question 48.
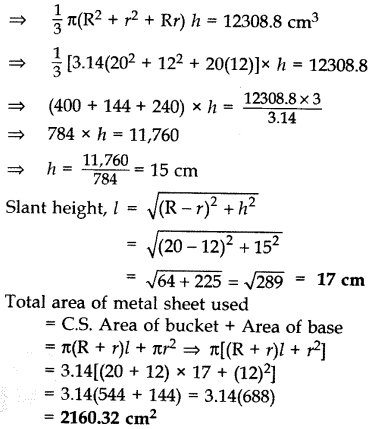
A bucket open at the top is in the form of a frustum of a cone with a capacity of 12308.8 cm³ The radii of the top and bottom circular ends are 20 cm and 12 cm respectively. Find the height of the bucket and the area of metal sheet used in making the bucket. (Use π = 3.14)
Year of Question: (2016D)

Solution:
Radius of the top of bucket, R = 20 cm
Radius of the bottom of bucket, r = 12 cm
Voulume of bucket = 12308.8 cm³

Question 49.
A container shaped like a right circular cylinder having base radius 6 cm and height 15 cm is full of ice-cream. The ice-cream is to be filled into cones of height 12 cm and radius 3 cm, having a hemispherical shape on the top. Find the number of such cones which can be filled with ice-cream.
Year of Question: (2012D)

Solution:
Volume of cylinder = πr²h
Volume of ice-cream cone = 1/3 πr²₁h₁
Volume of hemispherical top of ice-cream

Question 50.
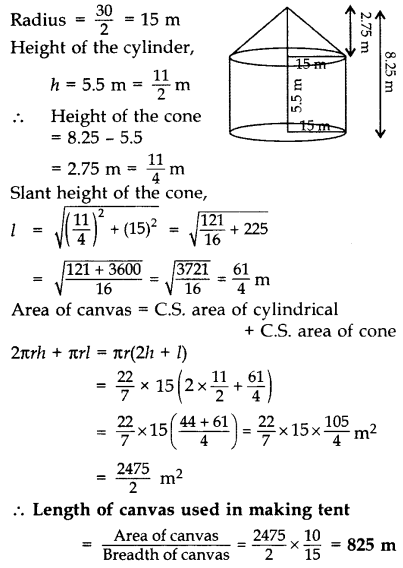
A military tent of height 8.25 m is in the form of a right circular cylinder of base diameter 30 m and height 5.5 m surmounted by a right circular cone of same base radius. Find the length of the canvas used in making the tent, if the breadth of the canvas is 1.5 m.
Year of Question: (2012OD)

Solution:

Question 51.
A well of diameter 4 m is dug 14 m deep. The earth taken out is spread evenly all around the well to form a 40 cm high embankment. Find the width of the embankment.
Year of Question: (2015D)

Solution:
Let h be the height of well and r be the radius of well.
h = 14 m, r= d/2=4/2 = 2 m
Volume of earth taken out after digging the well =πr²h
= (22/7×2×2×14) m³ = 176 m³ .(i)
Let x be the width of embankment formed by using (i),
∴ Total width of well including embankment (R) = 2 + x
Height of embankment (H) = 40 cm = 40/100 m
Volume of well = Volume of embankment So, Volume of embankment = T(R² - r²)H = 176 .[From (i)

Question 52.
A tent consists of a frustum of a cone, surmounted by a cone. If the diameter of the upper and lower circular ends of the frustum be 14 m and 26 m respectively, the height of the frustum be 8 m and the slant height of the surmounted conical portion be 12 m, find the area of canvas required to make the tent. (Assume that the radii of the upper circular end of the frustum and the base of surmounted conical portion are equal).
Year of Question: (2014OD)

Solution:
Upper radius of frustum of a cone,

Question 53.
Water is flowing at the rate of 15 km/hour through a pipe of diameter 14 cm into a cuboidal pond which is 50 m long and 44 m wide. In what time will the level of water in the pond rise by 21 cm?
Year of Question: (2011OD)

Solution:
H = 15 km = 15000 m (1 km = 1000 m)
Radius of pipe (t) = 142 = 7 cm = 7100 m
Volume of pipe (cylinder) =πr²H
∴ Volume of water flowing through the cylindrical pipe in an hour at the rate of 15 km/hr.

Question 54.
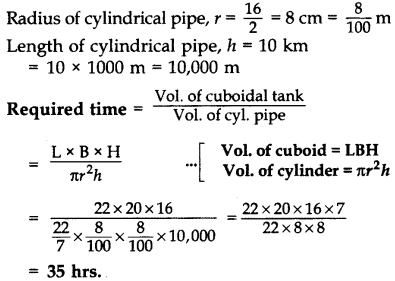
Water is flowing at the rate of 10 km/hour through a pipe of diameter 16 cm into a cuboidal tank of dimensions 22 m × 20 m × 16 m. How long will it take to fill the empty tank? [Use π = 22/7]
Year of Question: (2011OD)

Solution:

Question 55.
A drinking glass is in the shape of the frustum of a cone of height 14 cm. The diameters of its two circular ends are 4 cm and 2 cm. Find the capacity of the glass. [Use π = 22/7]
Year of Question: (2012OD)

Solution:

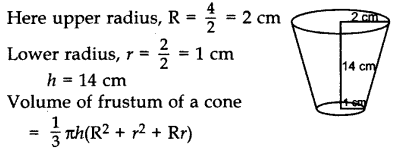
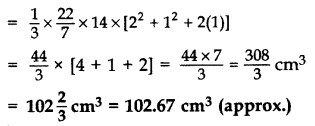
Question 56.
A bucket open at the top, and made up of a metal sheet is in the form of a frustum of a cone. The depth of the bucket is 24 cm and the diameters of its upper and lower circular ends are 30 cm and 10 cm respectively. Find the cost of metal sheet used in it at the rate of 10 per 100 cm². (Useπ = 3.14)
Year of Question: (2013D)

Solution:
Depth of bucket, h = 24 cm
Radius of top bucket,

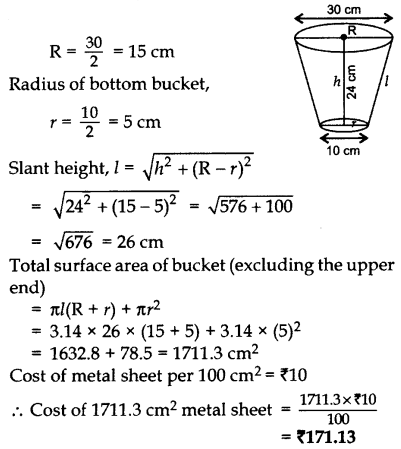
Question 57.
The slant height of a frustum of a cone is 4 cm and the perimeters of its circular ends are 18 cm and 6 cm. Find the curved surface area of the frustum.
Year of Question: (2017OD)

Solution:
Let longer radius = R cm
Smaller radius = r cm
2πR = 18 and 2πr = 6

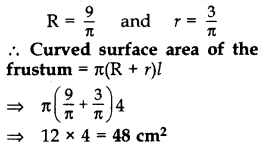
Question 58.
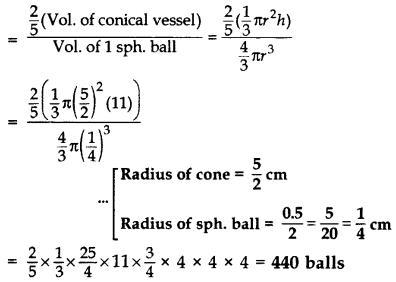
Sushant has a vessel, of the form of an inverted cone, open at the top, of height 11 cm and radius of top as 2.5 cm and is full of water. Metallic spherical balls each of diameter 0.5 cm are put in the vessel due to which 2/5 th of the water in the vessel flows out. Find how many balls were put in the vessel. Sushant made the arrangement so that the water that flows out irrigates the flower beds.
Year of Question: (2014D)

Solution:
Numbers of spherical balls

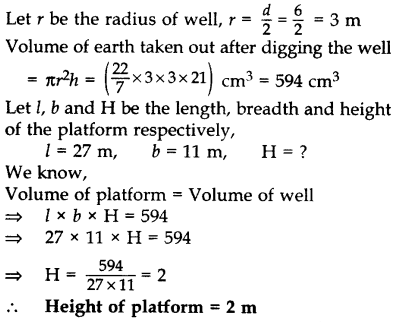
Question 59.
A 21 m deep well with diameter 6 m is dug and the earth from digging is evenly spread to form a platform 27 m × 11 m. Find the height of the platform. (Useπ = 22/7]
Year of Question: (2015D)

Solution:
Let h be the height of well, h = 21 m

Question 60.
The dimensions of a solid iron cuboid are 4.4 m × 2.6 m × 1.0 m. It is melted and recast into a hollow cylindrical pipe of 30 cm inner radius and thickness 5 cm. Find the length of the pipe.
Year of Question: (2017OD)

Solution:

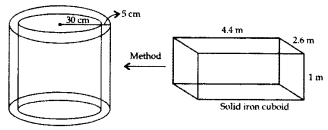
Inner radius, r = 30 cm = 0.30 m
Outer radius, R = (30 + 5) cm = 35 cm or 0.35 m
As per the question: (Volume of hollow Cylindrical pipe) = (Volume of solid iron cuboid)

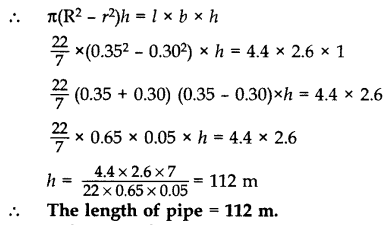
Question 61.
In a rain-water harvesting system, the rain water from a roof of 22 m × 20 m drains into a cylindrical tank having diameter of base 2 m and height 3.5 m. If the tank is full, find the rainfull in cm.
Year of Question: (2017OD)

Solution:
Let the rainfall be x m.
Volume of water on the roof = Volume of cylindrical tank

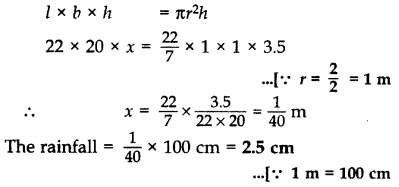
Question 62.
A bucket is in the form of a frustum of a cone and it can hold 28.49 litres of water. If the radii of its circular ends are 28 cm and 21 cm, find the height of the bucket. (Use π = 22/7)
Year of Question: (2012D)

Solution:
Here R = 28 cm, r = 21 cm
Volume of Bucket = 28.49 litres

Question 63.
Water running in a cylindrical pipe of inner diameter 7 cm, is collected in a container at the rate of 192.5 litres per minute. Find the rate of flow of water in the pipe in km/h. (Use π= 22/7)
Year of Question: (2013OD)

Solution:
Volume of water that flows for one hour
= (192.50 × 60) Its.
= 192.50 × 60 × 1000 cm³ .[∵ 1 lt. 1000 cm³
Inner radius of the cylindrical pipe = 7/2 cm
Let h cm be the length of the volume of water that flows in one hour.
Volume of water that flows in 1 hr. = πr²h
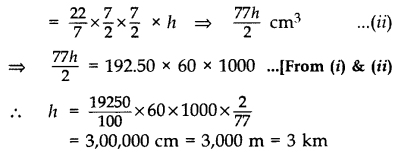Hence the rate of flow of water in the pipe = 3 km/hr.

Question 64.
Due to heavy floods in a State, thousands were rendered homeless. 50 schools collectively offered to the State Government to provide place and the canvas for 1,500 tents to be fixed by the Government and decided to share the whole expenditure equally. The lower part of each tent is cylindrical of base radius 2.8 m and height 3.5 m, with conical upper part of same base radius but of height 2.1 m. If the canvas used to make the tents costs ₹120 per sq. m, find the amount shared by ‘each school to set up the tents.
Year of Question: (2016OD)

Solution:

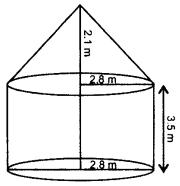
Let r and h be the radius and height of cylindrical part respectively and I be the slant height of conical part.
Slant height of conical part (l),

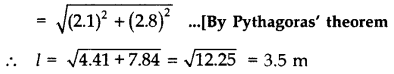
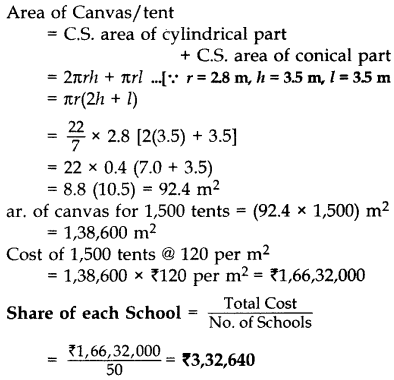
Question 65.
A container, open at the top and made up of metal sheet is in the form of a frustum of a cone of height 16 cm with diameters of its lower and upper ends as 16 cm and 40 cm respectively. Find the cost of metal sheet used to make the container, if it costs 10 per 100 cm². [Take a = 3.14]
Year of Question: (2013OD)

Solution:
Height of frustum of a cone, h = 16 cm

• CBSE Class 10 SA-1 Mathematics (Basics) Set 1 QP
• CBSE Class 10 Maths Surface Areas MCQ