NCERT CBSE for Class 10 Maths Chapter 6 Triangles Important Questions
Triangles Class 10 Important Questions Very Short Answer (1 Mark)
Question 1.
If ∆ABC ~ ∆PQR, perimeter of ∆ABC = 32 cm, perimeter of ∆PQR = 48 cm and PR = 6 cm, then find the length of AC.
Year of Question:(2012)
Solution:
∆ABC ~ ∆PQR .[Given

Question 2.
∆ABC ~ ∆DEF. If AB = 4 cm, BC = 3.5 cm, CA = 2.5 cm and DF = 7.5 cm, find the perimeter of ∆DEF.
Year of Question:(2012, 2017D)
Solution:
∆ABC - ∆DEF .[Given
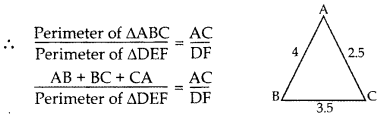
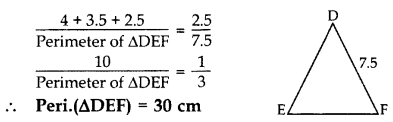
Question 3.
If ∆ABC ~ ∆RPQ, AB = 3 cm, BC = 5 cm, AC = 6 cm, RP = 6 cm and PQ = 10, then find QR.
Year of Question: (2014)
Solution:
∆ABC ~ ∆RPQ .[Given

∴ QR = 12 cm
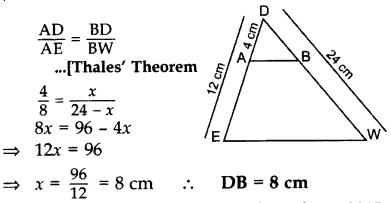
Question 4.
In ∆DEW, AB ‖ EW. If AD = 4 cm, DE = 12 cm and DW = 24 cm, then find the value of DB.
Year of Question: (2015)
Solution:
Let BD = x cm
then BW = (24 - x) cm, AE = 12 - 4 = 8 cm
In ∆DEW, AB ‖ EW
Question 5.
In ∆ABC, DE ‖ BC, find the value of x.
Year of Question: (2015)
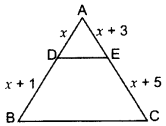
Solution:
In ∆ABC, DE ‖ BC .[Given
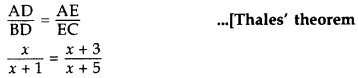
x(x + 5) = (x + 3)(x + 1)
x2 + 5x = x2 + 3x + x + 3
x2 + 5x - x2 - 3x - x = 3
∴ x = 3 cm
Question 6.
In the given figure, if DE ‖ BC, AE = 8 cm, EC = 2 cm and BC = 6 cm, then find DE.
Year of Question: (2014)
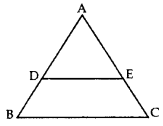
Solution:
In ∆ADE and ∆ABC,
∠DAE = ∠BAC .Common
∠ADE - ∠ABC . [Corresponding angles
∆ADE - ∆??C .[AA corollary
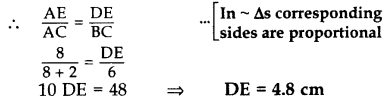
Question 7.
In the given figure, XY ‖ QR, PQ/XQ=7/3 and PR = 6.3 cm, find YR.
Year of Question: (2017OD)

Solution:
Let YR = x
PQ/XQ=PR/YR . [Thales’ theorem
Question 8.
The lengths of the diagonals of a rhombus are 24 cm and 32 cm. Calculate the length of the altitude of the rhombus.
Year of Question: (2013)
Solution:
Diagonals of a rhombus are ⊥ bisectors of each other.
∴ AC ⊥ BD,
OA = OC = AC2⇒242 = 12 cm
OB = OD = BD2⇒322 = 16 cm
In rt. ∆BOC,
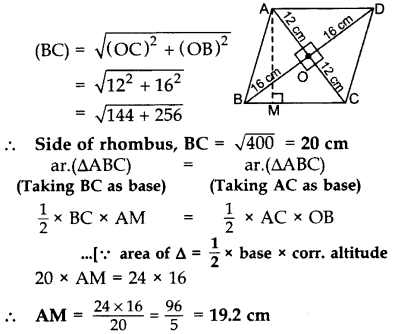
Question 9.
If PQR is an equilateral triangle and PX ⊥ QR, find the value of PX2.
Year of Question: (2013)
Solution:
Altitude of an equilateral ∆,
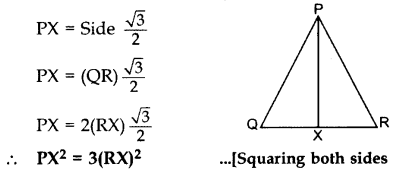
Triangles Class 10 Important Questions Short Answer-I (2 Marks)
Question 10.
The sides AB and AC and the perimeter P, of ∆ABC are respectively three times the corresponding sides DE and DF and the perimeter P, of ∆DEF. Are the two triangles similar? If yes, find ar(∆ABC)ar/(∆DEF)
Year of Question: (2012)
Solution:
Given: AB = 3DE and AC = 3DF

.[∵ The ratio of the areas of two similar ∆s is equal to the ratio of the squares of their corresponding sides
Question 11.
In the figure, EF ‖ AC, BC = 10 cm, AB = 13 cm and EC = 2 cm, find AF.
Year of Question: (2014)
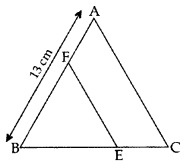
Solution:
BE = BC - EC = 10 - 2 = 8 cm
Let AF = x cm, then BF = (13 - x) cm
In ∆ABC, EF‖AC . [Given
Question 12.
X and Y are points on the sides AB and AC respectively of a triangle ABC such that AX/AB=1/4, AY = 2 cm and YC = 6 cm. Find whether XY ‖BC or not.
Year of Question: (2015)
Solution:.
Given: AX/AB=1/4

AX = 1K, AB = 4K
∴ BX = AB - AX
= 4K - 1K = 3K
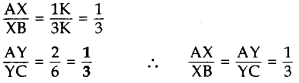
∴ XY ‖BC . [By converse of Thales’ theorem
Question 13.
In the given figure, ∠A = 90°, AD ⊥ BC. If BD = 2 cm and CD = 8 cm, find AD.
Year of Question:(2012; 2017D)
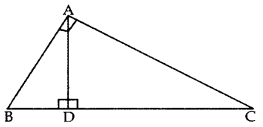
Solution:
∆ADB ~ ∆CDA .[If a perpendicular is drawn from the vertex of the right angle of a rt. ∆ to the hypotenuse then As on both sides of the ? are similar to the whole D and to each other
∴ BD/AD=AD/CD .[ ∵ Sides are proportional
AD2 = BD , DC
AD2 = (2) (8) = 16 ⇒ AD = 4 cm
Question 14.
In ∆ABC, ∠BAC = 90° and AD ⊥ BC. Prove that ADfrac{B D}{A D}=frac{A D}{C D} = BD × DC.
Year of Question:(2013)
Solution:
In 1t. ∆BDA, ∠1 + ∠5 = 90°
In rt. ∆BAC, ∠1 + ∠4 = 90° .(ii)
∠1 + ∠5 = ∠1 + ∠4 .[From (i) & (ii)
.. ∠5 = ∠4 .(iii)
In ∆BDA and ∆ADC,
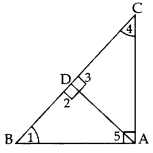
∠5 = 24 . [From (iii)
∠2 = ∠3 .[Each 90°
∴ ∆BDA ~ ∆ADC.[AA similarity
BD/AD=AD/CD
. [In ~ As corresponding BA sides are proportional
∴ AD
2 = BD × DC
Question 15.
A 6.5 m long ladder is placed against a wall such that its foot is at a distance of 2.5 m from the wall. Find the height of the wall where the top of the ladder touches it.
Year of Question:(2015)
Solution:
Let AC be the ladder and AB be the wall.
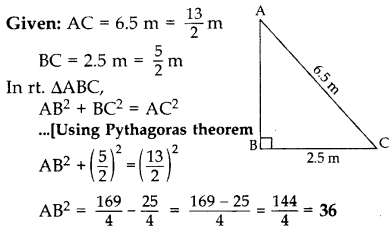
∴Required height, AB = 6 m
Question 16.
In the figure ABC and DBC are two right triangles. Prove that AP × PC = BP × PD.
Year of Question:(2013)
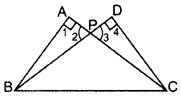
Solution:
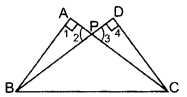
In ∆APB and ∆DPC,
∠1 = ∠4 . [Each = 90°
∠2 = ∠3 .[Vertically opp. ∠s
∴ ∆APB ~ ∆DPC .[AA corollary
⇒ BP/PC=AP/PD . [Sides are proportional
∴ AP × PC = BP × PD
Question 17.
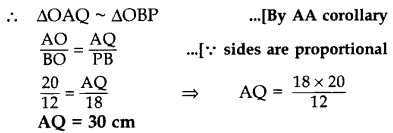
In the given figure, QA ⊥ AB and PB ⊥ AB. If AO = 20 cm, BO = 12 cm, PB = 18 cm, find A Question
Year of Question:(2017OD)

Solutions
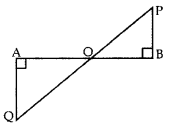
In ∆OAQ and ∆OBP,
∠OAQ = ∠OBP . [Each 90°
∠AOQ = ∠BOP . [vertically opposite angles
Triangles Class 10 Important Questions Short Answer-II (3 Marks)
Question 18.
In the given figure, CD ‖ LA and DE ‖ AC. Find the length of CL if BE = 4 cm and EC = 2 cm.
Year of Question:(2012)
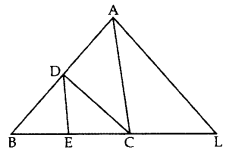
Solutions
In ∆ABL, CD ‖ LA

Question 19.
If a line segment intersects sides AB and AC of a ∆ABC at D and E respectively and is parallel to BC, prove that AD/AB=AE/AC.
Year of Question:(2013)
Solution:
Given. In ∆ABC, DE ‖ BC
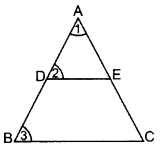
To prove. AD/AB=AE/AC
Proof.
In ∆ADE and ∆ABC
∠1 = ∠1 . Common
∠2 = ∠3 . [Corresponding angles
∆ADE ~ ∆ABC .[AA similarity
∴ AD/AB=AE/AC
.[In ~∆s corresponding sides are proportional
Question 20.
In a ∆ABC, DE ‖ BC with D on AB and E on AC. If AD/DB=34 , find BC/DE.
Year of Question:(2013)
Solution:
Given: In a ∆ABC, DE ‖BC with D on AB and E
on AC and AD/DB=34
To find: BC/DE
Proof. Let AD = 3k,

DB = 4k
∴ AB = 3k + 4k = 7k
In ∆ADE and ∆ABC,
∠1 = ∠1 .[Common
∠2 = ∠3 . [Corresponding angles
∴∆ADE ~∆ABC .[AA similarity

Question 21.
In the figure, if DE ‖ OB and EF ‖ BC, then prove that DF ‖ OC.
Year of Question:(2014)
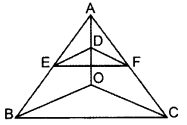
Solution:
Given. In ∆ABC, DE ‖ OB and EF ‖ BC
To prove. DF ‖OC
Proof. In ∆AOB, DE ‖OB . [Given
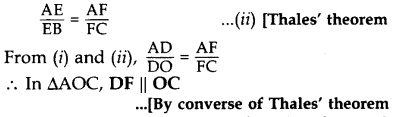
Question 22.
If the perimeters of two similar triangles ABC and DEF are 50 cm and 70 cm respectively and one side of ∆ABC = 20 cm, then find the corresponding side of ∆DEF.
Year of Question:(2014)
Solution:
Given. ∆ABC ~ ∆DEF,
Perimeter(∆ABC) = 50 cm
Perimeter(∆DEF) = 70 cm
One side of ∆ABC = 20 cm
To Find. Corresponding side of ∆DEF (i.e.,) DE. ∆ABC ~ ∆DEF .[Given

∴ The corresponding side of ADEF = 28 cm
Question 23.
A vertical pole of length 8 m casts a shadow 6 cm long on the ground and at the same time a tower casts a shadow 30 m long. Find the height of tower.
Year of Question:(2014)
Solution:
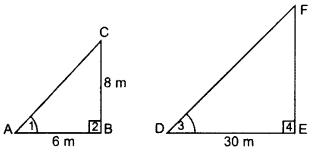
Let BC be the pole and EF be the tower Shadow AB = 6 m and DE = 30 m.
In ∆ABC and ∆DEF,
∠2 = ∠4 . [Each 90°
∠1 = ∠3 . [Sun’s angle of elevation at the same time
∆ABC ~ ∆DEF .[AA similarity
AB/DE=BC/EF . [In -As corresponding sides are proportional
⇒ 630=8EF ∴ EF = 40 m
Question 24.
In given figure, EB ⊥ AC, BG ⊥ AE and CF ⊥ AE
Year of Question:(2015)
Prove that:
(a) ∆ABG ~ ∆DCB
(b) BC/BD=BE/BA
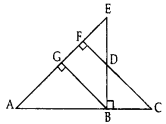
Solution:
Given: EB ⊥ AC, BG ⊥ AE and CF ⊥ AE.
To prove: (a) ∆ABG - ∆DCB,
(b) BC/BD=BE/BA
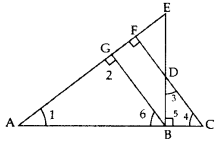
Proof: (a) In ∆ABG and ∆DCB,
∠2 = ∠5 . [each 90°
∠6 = ∠4 . [corresponding angles
∴ ∆ABG ~ ∆DCB . [By AA similarity
(Hence Proved)
∴ ∠1 = ∠3 .(CPCT . [In ~∆s, corresponding angles are equal
(b) In ∆ABE and ∆DBC,
∠1 = ∠3 .(proved above
∠ABE = ∠5 . [each is 90°, EB ⊥ AC (Given)
∆ABE ~ ∆DBC . [By AA similarity
BC/BD=BE/BA
. [In ~∆s, corresponding sides are proportional
∴BC/BD=BE/BA (Hence Proved)
Question 25.
∆ABC ~∆PQR. AD is the median to BC and PM is the median to QR. Prove that AB/PQ=AD/PM.
Year of Question:(2017D)
Solution:
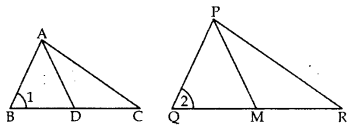
∆ABC ~∆PQR . [Given
∠1 = ∠2 . [In ~∆s corresponding angles are equal
Question 26.
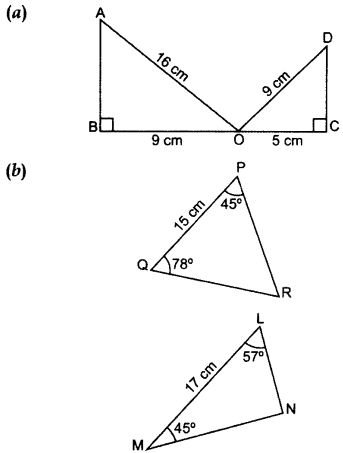
State whether the given pairs of triangles are similar or not. In case of similarity mention the criterion.
Year of Question:(2015)
Solution:

(b) In ∆PQR, ∠P + ∠Q + ∠ZR = 180° .[Angle-Sum Property of a ∆
45° + 78° + ∠R = 180°
∠R = 180° - 45° - 78° = 57°
In ∆LMN, ∠L + ∠M + ∠N = 180° .[Angle-Sum Property of a ∆
57° + 45° + ∠N = 180°
∠N = 180° - 57 - 45° = 78°
∠P = ∠M . (each = 45°
∠Q = ∠N . (each = 78°
∠R = ∠L .(each = 57°
∴ ∆PQR - ∆MNL .[By AAA similarity theorem
Question 27.
In the figure of ∆ABC, D divides CA in the ratio 4 : 3. If DE‖ BC, then find ar (BCDE) : ar (∆ABC).
Year of Question:(2015)
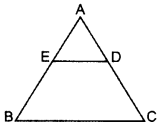
Solution:
Given:
D divides CA in 4 : 3
CD = 4K
DA = 3K
DE ‖ BC .[Given
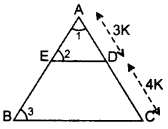
In ∆AED and ∆ABC,
∠1 = ∠1 .[common
∠2 = ∠3 . corresponding angles
∴ ∆AED - ∆ABC .(AA similarity
⇒ ar(∆AED)/ar(∆ABC)=(AD/AC)2
. [The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides
⇒ frac(3K)²(7K)²=9K²49K²=ar(∆AED)ar(∆ABC)=949
Let ar(∆AED) = 9p
and ar(∆ABC) = 49p
ar(BCDE) = ar (∆ABC) - ar (∆ADE)
= 49p - 9p = 40p
∴ ar(BCDE)/ar(∆ABC)=40p/49p
∴ ar (BCDE) : ar(AABC) = 40 : 49
Question 28.
In the given figure, DE‖BC and AD : DB = 7 : 5, find frac { arleft(riangle DEF
ight) }{ arleft(riangle CFB
ight) } [/latex]
Year of Question:(2017OD)

Solution:
Given: In ∆ABC, DE ‖ BC and AD : DB = 7 : 5.
To find: ar(∆DEF)ar(∆CFB) = ?
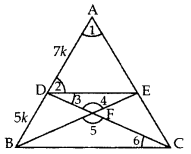
Proof: Let AD = 7k
and BD = 5k then
AB = 7k + 5k = 12k
In ∆ADE and ∆ABC,
∠1 =∠1.(Common
∠2 =∠ABC. [Corresponding angles
Question 29.
In the given figure, the line segment XY is parallel to the side AC of ∆ABC and it divides the triangle into two parts of equal areas. Find the ratio AX/AB.
Year of Question:(2017OD)
Solution:
We have XY ‖ AC . [Given
So, ∠BXY = ∠A and ∠BYX = ∠C .[Corresponding angles
∴ ∆ABC ~ ∆XBY .[AA similarity criterion

Question 30.
In the given figure, AD ⊥ BC and BD = 1/3 CD. Prove that 2AC2 = 2AB2 + BC2.
Year of Question:(2012)
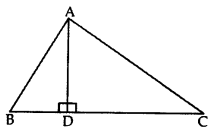
Solution:
BC = BD + DC = BD + 3BD = 4BD
∴ BC4 = BD
In rt. ∆ADB, AD2 = AB2 - BD2 ..(ii)
In rt. ∆ADC, AD2 = AC2 - CD2 .(iii)
From (ii) and (iii), we get
AC2 - CD2 = AB2 - BD2
AC2 = AB2 - BD2 + CD2
∴ 2AC2 = 2AB2 + BC2 (Hence proved)
Question 31.
In the given figure, ∆ABC is right-angled at C and DE ⊥ AB. Prove that ∆ABC ~ ∆ADE and hence find the lengths of AE and DE.
Year of Question:(2012, 2017D)
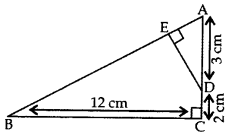
Solution:
Given: ∆ABC is rt. ∠ed at C and DE ⊥ AB.
AD = 3 cm, DC = 2 cm, BC = 12 cm
To prove:
(i) ∆ABC ~ ∆ADE; (ii) AE = ? and DE = ?
Proof. (i) In ∆ABC and ∆ADE,
∠ACB = ∠AED . [Each 90°
∠BAC = ∠DAE .(Common .
∴ ∆ABC ~ ∆ADE .[AA Similarity Criterion
(ii) ∴AB/AD=BC/DE=AC/AE . [side are proportional
AB/3=12/DE=3+2/AE
...[In rt. ∆ACB, . AB
2 = AC
2 + BC
2(By Pythagoras’ theorem)
= (5)
2 + (12)
2 = 169
∴AB = 13 cm
Question 32.
In ∆ABC, if AP ⊥ BC and AC2= BC2 - AB2, then prove that PA2 = PB × CP.
Year of Question:(2015)
Solution:

AC2 = BC2 - AB2 .Given
AC2 + AB2 = BC2
∴ ∠BAC = 90° . [By converse of Pythagoras’ theorem
∆APB ~ ∆CPA
[If a perpendicular is drawn from the vertex of the right angle of a triangle to the hypotenuse then As on both sides of the perpendicular are similar to the whole triangle and to each other.
∴ AP/CP=PB/PA . [In ~ ∆s, corresponding sides are proportional
∴ PA2 = PB. CP (Hence Proved)
Question 33.
ABCD is a rhombus. Prove that AB2 + BC2 + CD2 + DA2= AC2 + BD2.
Year of Question:(2013)
Solution:
Given. In rhombus ABCD, diagonals AC and BD intersect at O.
To prove: AB2 + BC2 + CD2 + DA2 = AC2 + BD2
Proof: AC ⊥ BD [∵ Diagonals of a rhombus bisect each other at right angles
∴ OA = OC and
OB = OD
In rt. ∆AOB,
AB2 = OA2 + OB2 . [Pythagoras’ theorem
AB2 = (AC/2)2+(BD/2)2
AB2 = (AC2)2+(BD2)2
4AB2 = AC2 + BD2
AB2 + AB2 + AB2 + AB2 = AC2 + BD2
∴ AB2 + BC2 + CD2 + DA2 = AC2 + BD2
.[∵ In a rhombus, all sides are equal
Question 34.
The diagonals of trapezium ABCD intersect each other at point o. If AB = 2CD, find the ratio of area of the ∆AOB to area of ∆COD.
Year of Question:(2013)
Solution:
In ∆AOB and ∆COD, . [Alternate int. ∠s
∠1 = ∆3
∠2 = ∠4
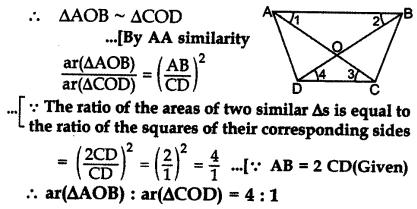
Question 35.
The diagonals of a quadrilateral ABCD intersect each other at the point O such that AO/BO=CO/DO. Show that ABCD is a trapezium.
Year of Question:(2014)
Solution:
1st method.
Given: Quadrilateral ABCD in which
AC and BD intersect each other at 0.
Such that AO/BO=CO/DO
To prove: ABCD is a trapezium
Const.: From O, draw OE ‖ CD.


But these are alternate interior angles
∴ AB ‖ DC Quad. ABCD is a trapezium.
Triangles Class 10 Important Questions Long Answer (4 Marks).
Question 36.
In a rectangle ABCD, E is middle point of AD. If AD = 40 m and AB = 48 m, then find EB.
Year of Question:(2014D)
Solution:
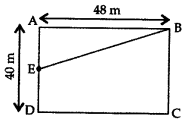
E is the mid-point of AD .[Given
AE = 40/2 = 20 m
∠A = 90° .[Angle of a rectangle
In rt. ∆BAE,
EB2 = AB2 + AE2 .[Pythagoras’ theorem
= (48)2 + (20)2
= 2304 + 400 = 2704
∴ EB = √2704= 52 m
Question 37.
Let ABC be a triangle and D and E be two points on side AB such that AD = BE. If DP ‖BC and EQ ‖AC, then prove that PQ ‖AB.
Year of Question:(2013)
Solution:
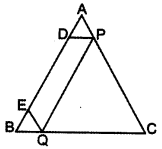
In ∆ABC,
DP ‖ BC
and EQ ‖AC . [Given
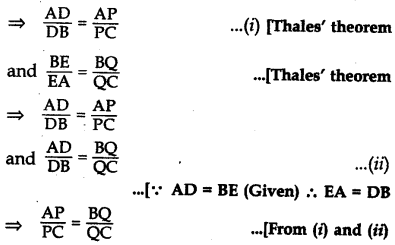
Now, in ∆ABC, P and Q divide sides CA and CB respectively in the same ratio.
∴ PQ ‖ AB
Question 38.
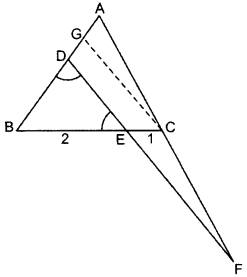
In the figure, ∠BED = ∠BDE & E divides BC in the ratio 2 : 1.
Prove that AF × BE = 2 AD × CF.
Year of Question:(2015)
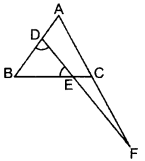
Solution:
Construction:
Draw CG‖ DF
Proof: E divides
BC in 2 : 1.
BE/EC=2/1 .(i)
Question 39.
In the given figure, AD = 3 cm, AE = 5 cm, BD = 4 cm, CE = 4 cm, CF = 2 cm, BF = 2.5 cm, then find the pair of parallel lines and hence their lengths.
Year of Question:(2015)
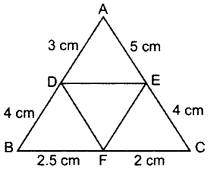
Solution:
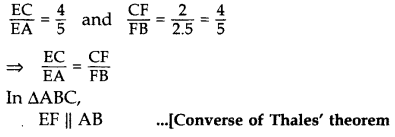
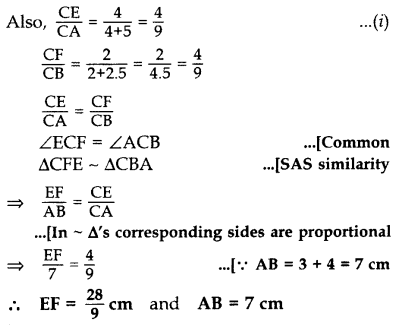
Question 40.
If sides AB, BC and median AD of AABC are proportional to the corresponding sides PQ, QR and median PM of PQR, show that ∆ABC ~ ∆PQR.
Year of Question:(2017OD)
Solution:
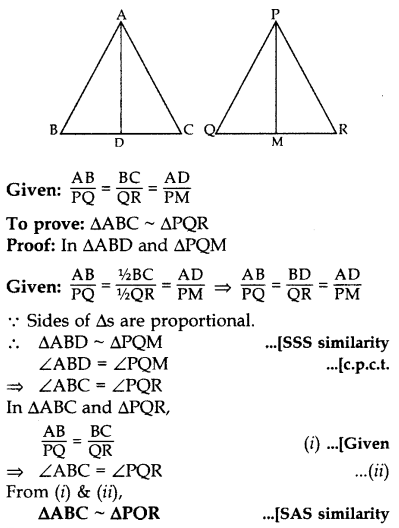
Question 41.
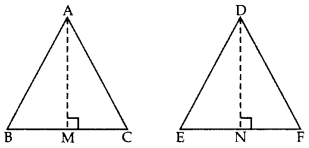
Prove that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
Year of Question:(2012)
Solution:
Given: ∆ABC ~ ∆DEF


Question 42.
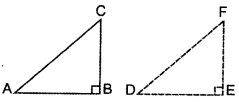
State and prove converse of Pythagoras theorem. Using the above theorem, solve the following: In ∆ABC, AB = 63 cm, BC = 6 cm and AC = 12 cm, find ∠B.
Year of Question:(2015)
Solution:
Part I:
Statement: Prove that, in a triangle, if square of one side is equal to the sum of the squares of the other two sides, then the angle opposite the first side is a right angle.
To prove: ∠ABC = 90°
Const.: Draw a right angle ∆DEF in which DE = BC and EF = AB.
Proof: In rt. ∆ABC,
AB2 + BC2 = AC2 .(i) Given
In rt. ∆DEF
DE2 + EF2 = DF2 . [By Pythagoras’ theorem
BC2 + AB2 = DF2.(ii).[? DE = BC; EF = AB
From (i) and (ii), we get
AC2 = DF2 = AC = DF
Now, DE = BC .[By construction
EF = AB .[By construction
DF = AC . [Proved above :
∴ ∆DEF = ∆ABC . (SSS congruence :
∴ ∠DEF = ∠ABC .[c.p.c.t.
∡DEF = 90° ∴ ∠ABC = 90°
Given: In rt. ∆ABC,
AB2 + BC2 = AC2
AB2+ BC2 = (6√3)2 + (6)2
= 108 + 36 = 144 = (12)2
AB2 + BC2 = AC2 ∴ ∠B = 90° . [Above theorem
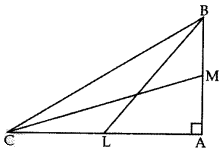
Question 43.
In the given figure, BL and CM are medians of a triangle ABC, right angled at A. Prove that: 4(BL2 + CM2) = 5BC2
Year of Question:(2012)
Solution:
Given: BL and CM are medians of ?ABC, right angled at A.
To prove: 4(BL2 + CM2) = 5 BC2
Proof: In ∆ABC, BC2 = BA2 + CA2 .(i)
In ∆BAL,
BL2 = BA2 + AL2 .[Pythagoras̱ theorem
BL2 = BA2 + (CA/2)2
BL2 = BA2+ CA2/4
⇒ 4BL2 = 4BA2 + CA2 .(ii)
Now, In ∆MCA,
MC2 = CA2 + MA2 .[Pythagoras̱ theorem
MC2 = CA22 + (BA2)/2
MC2 = CA2 + BA2/4
4MC2 = 4CA2 + BA2
Adding (ii) and (iii), we get
4BL2 + 4MC2 = 4BA2 + CA2 + 4CA2+ BA2 .[From (ii) & (iii)
4(BL2 + MC2) = 5BA2 + 5CA2
4(BL2 + MC2) = 5(BA2 + CA2)
∴ 4(BL2 + MC2) = 5BC2 . [Using (1)
Hence proved.
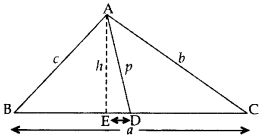
Question 44.
In the given figure, AD is median of ∆ABC and AE ⊥ BC.
Year of Question:(2013)
Prove that b2+ c2 = 2p2 + 1/2 a2.
Solutions
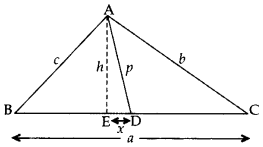
Proof. Let ED = x
BD = DC = BC/2=a/2 = .[∵ AD is the median
In rt. ∆AEC, AC² = AE² + EC² ...[By Pythagoras’ theorem
b² = h² + (ED + DC)²
b² = (p² - x²) + (x = a/2)²
.[∵ In rt. ∆AED, x² + h² = p² ⇒ h² = p² - x².(i)
b2 = p² - x² + x² + (a/2)²2+ 2(x)(a²)
b2 = p² + ax + a²/4 .(ii)
In rt. ∆AEB, AB² = AE²+ BE². [By Pythagoras’theorem
Question 45.
In a ∆ABC, the perpendicular from A on the side BC of a AABC intersects BC at D such that DB = 3 CD. Prove that 2 AB² = 2 AC² + BC².
Year of Question:(2013; 2017OD)
Solution:
In rt. ∆ADB,
AD² = AB² - BD².(i) [Pythagoras̱ theorem
In rt. ∆ADC,
AD2= AC2 - DC2 .(ii) [Pythagoras̱ theorem
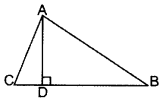
From (i) and (ii), we get
AB² - BD² = AC² - DC²
AB² = AC² + BD²- DC²
Now, BC = BD + DC
= 3CD + CD = 4 CD .[∵ BD = 3CD (Given)
⇒ BC² = 16 CD² .(iv) [Squaring
Now, AB² = AC² + BD² - DC² .[From (iii)
= AC² + 9 DC² - DC² ..[∵ BD = 3 CD ⇒ BD² = 9 CD²
= AC²+ 8 DC²
= AC² + 16DC²/2
= AC² + BC²/2 . [From (iv)
∴ 2AB² = 2AC² + BC² . [Proved
Question 46.
In ∆ABC, altitudes AD and CE intersect each other at the point P. Prove that:
Year of Question:(2014)
(i) ∆ APE ~ ∆CPD
(ii) AP × PD = CP × PE
(iii) ∆ADB ~ ∆CEB
(iv) AB × CE = BC × AD
Solution:
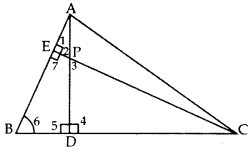
Given. In ∆ABC, AD ⊥ BC & CE ⊥ AB.
To prove. (i) ∆APE ~ ∆CPD
(ii) AP × PD = CP × PE
(iii) ∆ADB ~ ∆CEB
(iv) AB × CE = BC × AD
Proof: (i) In ∆APE and ∆CPD,
∠1 = ∠4 .[Each 90°
∠2 = ∠3 .[Vertically opposite angles
∴ ∆APE ~ ∆CPD .[AA similarity
(ii) AP/CP=PE/PD . [In ~ ∆s corresponding sides are proportional
∴ AP × PD = CP × PE
(iii) In ∆ADB and ∆CEB,
∠5 = ∠7 .[Each 90°
∠6 = ∠6 .(Common
∴ ∆ADB ~ ∆CEB .[AA similarity
(iv) ∴ AB/CB=AD/CE . [In ~ ∆s corresponding sides are proportional
∴ AB × CE = BC × AD
Question 47.
In the figure, PQR and QST are two right triangles, right angled at R and T resepctively. Prove that QR × QS = QP × QT.
Year of Question:(2014)

Solution:
Given: Two rt. ∆‘s PQR and QST.
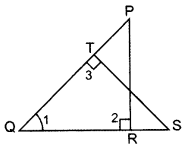
To prove: QR × QS = QP × QT
Proof: In ∆PRQ and ∆STQ,
∠1 = ∠1 . [Common
∠2 = ∠3 . [Each 90°
∆PRQ ~ ∆STO .(AA similarity
∴ QR/QT=QP/QS ..[In -∆s corresponding sides are proportional
∴ QR × QS = QP × QT (Hence proved)
Question 48.
In the given figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)/ar(DBC)=AO/DO.
Year of Question:(2012)
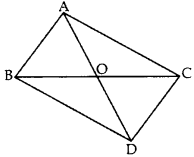
Solution:
Given: ABC and DBC are two As on the same base BC. AD intersects BC at O.
To prove:
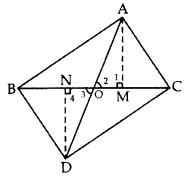
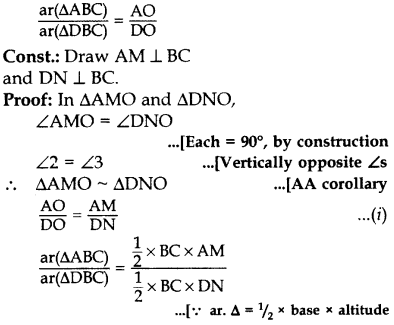

Question 49.
Hypotenuse of a right triangle is 25 cm and out of the remaining two sides, one is longer than the other by 5 cm. Find the lengths of the other
two sides.
Year of Question:(2013)
Solution:
Let Base, AB = x cm
Then altitude, BC = (x + 5) cm
In rt. ∆,
By Pythagoras’theorem
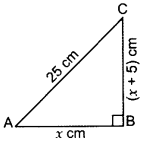
AB²+ BC² = AC²
⇒ (x)² + (x + 5)² = 25²
⇒ x²2 + x² + 10x + 25 - 625 = 0
⇒ 2x² + 10x - 600 = 0
⇒ x² + 5x - 300 = 0 . [Dividing both sides by 2
⇒ x² + 20x - 15x - 300 = 0
⇒ x(x + 20) - 15(x + 20) = 0
(x - 15)(x + 20) = 0
x - 15 = 0 or x + 20 = 0
x = 15 or x = -20
Base cannot be -ve
∴ x = 15 cm
∴ Length of the other side = 15 + 5 = 20 cm
Two sides are = 15 cm and 20 cm
Question 50.
In Figure, AB ⊥ BC, FG ⊥ BC and DE ⊥ AC. Prove that ∆ADE ~ ∆GCF.
Year of Question:(2016OD)
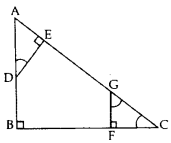
Solution:
In rt. ∆ABC,
∠A + ∠C = 90° .(i)
In rt. ∆AED,
∠A + ∠2 = 90°
From (i) and (ii), ∠C = ∠2
Similarly, ∠A = ∠1
Now in ∆ADE & ∆GCF
∠A = 1 . [Proved
∠C = 2 . [Proved
∠AED = ∠GFC . [rt. ∠s
∴ ∆ADE - ∆GCF .(Hence Proved)